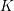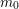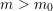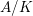Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Várilly-Alvarado, Anthony
2017.
Geometry Over Nonclosed Fields.
p.
197.
VÁRILLY-ALVARADO, ANTHONY
and
VIRAY, BIANCA
2017.
ABELIAN -DIVISION FIELDS OF ELLIPTIC CURVES AND BRAUER GROUPS OF PRODUCT KUMMER & ABELIAN SURFACES.
Forum of Mathematics, Sigma,
Vol. 5,
Issue. ,
Abramovich, Dan
and
Várilly-Alvarado, Anthony
2018.
Level structures on Abelian varieties, Kodaira dimensions, and Lang's conjecture.
Advances in Mathematics,
Vol. 329,
Issue. ,
p.
523.
Ellenberg, Jordan S.
Satriano, Matthew
and
Zureick-Brown, David
2023.
Heights on stacks and a generalized Batyrev–Manin–Malle conjecture.
Forum of Mathematics, Sigma,
Vol. 11,
Issue. ,
Box, Josha
and
Le Fourn, Samuel
2023.
Bounding integral points on the Siegel modular variety $$A_2(2)$$.
Research in Number Theory,
Vol. 9,
Issue. 2,