Published online by Cambridge University Press: 17 February 2020
Fix 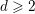 $d\geqslant 2$ and a field
$d\geqslant 2$ and a field 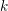 $k$ such that
$k$ such that 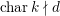 $\operatorname{char}k\nmid d$. Assume that
$\operatorname{char}k\nmid d$. Assume that 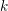 $k$ contains the
$k$ contains the 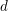 $d$th roots of
$d$th roots of 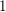 $1$. Then the irreducible components of the curves over
$1$. Then the irreducible components of the curves over 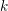 $k$ parameterizing preperiodic points of polynomials of the form
$k$ parameterizing preperiodic points of polynomials of the form  $z^{d}+c$ are geometrically irreducible and have gonality tending to
$z^{d}+c$ are geometrically irreducible and have gonality tending to 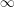 $\infty$. This implies the function field analogue of the strong uniform boundedness conjecture for preperiodic points of
$\infty$. This implies the function field analogue of the strong uniform boundedness conjecture for preperiodic points of 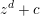 $z^{d}+c$. It also has consequences over number fields: it implies strong uniform boundedness for preperiodic points of bounded eventual period, which in turn reduces the full conjecture for preperiodic points to the conjecture for periodic points. Our proofs involve a novel argument specific to finite fields, in addition to more standard tools such as the Castelnuovo–Severi inequality.
$z^{d}+c$. It also has consequences over number fields: it implies strong uniform boundedness for preperiodic points of bounded eventual period, which in turn reduces the full conjecture for preperiodic points to the conjecture for periodic points. Our proofs involve a novel argument specific to finite fields, in addition to more standard tools such as the Castelnuovo–Severi inequality.
The second author was supported in part by National Science Foundation grants DMS-1069236 and DMS-1601946 and Simons Foundation grants #402472 (to Bjorn Poonen) and #550033.