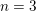Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hough, Robert
2019.
The shape of cubic fields.
Research in the Mathematical Sciences,
Vol. 6,
Issue. 3,
Hough, Bob
2019.
Equidistribution of bounded torsion CM points.
Journal d'Analyse Mathématique,
Vol. 138,
Issue. 2,
p.
765.
H, Piper
and
Harron, Robert
2020.
The shapes of Galois quartic fields.
Transactions of the American Mathematical Society,
Vol. 373,
Issue. 10,
p.
7109.
Mantilla-Soler, Guillermo
and
Rivera-Guaca, Carlos
2020.
A proof of a conjecture on trace-zero forms and shapes of number fields.
Research in Number Theory,
Vol. 6,
Issue. 3,
Bhargava, M.
Shankar, A.
Taniguchi, T.
Thorne, F.
Tsimerman, J.
and
Zhao, Y.
2020.
Bounds on 2-torsion in class groups of number fields and integral points on elliptic curves.
Journal of the American Mathematical Society,
Vol. 33,
Issue. 4,
p.
1087.
Harron, Robert
2021.
Equidistribution of shapes of complex cubic fields of fixed quadratic resolvent.
Algebra & Number Theory,
Vol. 15,
Issue. 5,
p.
1095.
Shankar, Ananth N.
Shankar, Arul
and
Wang, Xiaoheng
2021.
Large families of elliptic curves ordered by conductor.
Compositio Mathematica,
Vol. 157,
Issue. 7,
p.
1538.
Azpeitia Tellez, Fernando
Powell, Christopher
and
Sharif, Shahed
2021.
Geometry of biquadratic and cyclic cubic log-unit lattices.
Journal of Number Theory,
Vol. 228,
Issue. ,
p.
276.
Deopurkar, Anand
and
Patel, Anand
2022.
Vector bundles and finite covers.
Forum of Mathematics, Sigma,
Vol. 10,
Issue. ,
Hough, Robert
and
Lee, Eun Hye
2022.
Subconvexity of Shintani’s zeta function.
Transactions of the American Mathematical Society,
Vol. 375,
Issue. 11,
p.
8277.
Bolaños, Wilmar
and
Mantilla-Soler, Guillermo
2023.
The shape of cyclic number fields.
Canadian Mathematical Bulletin,
Vol. 66,
Issue. 2,
p.
599.
Shankar, Arul
and
Tsimerman, Jacob
2023.
Heuristics for the asymptotics of the number of Sn$S_n$‐number fields.
Journal of the London Mathematical Society,
Vol. 107,
Issue. 5,
p.
1613.
Ellenberg, Jordan S.
Satriano, Matthew
and
Zureick-Brown, David
2023.
Heights on stacks and a generalized Batyrev–Manin–Malle conjecture.
Forum of Mathematics, Sigma,
Vol. 11,
Issue. ,