Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-24T17:22:33.131Z Has data issue: false hasContentIssue false
  • English
  • Français

The elliptic Weyl character formula

Part of: Topological $K$-theory Lie groups Homology and cohomology theories

Published online by Cambridge University Press:  12 May 2014

Nora Ganter
Nora Ganter*
Affiliation:
Department of Mathematics and Statistics, The University of Melbourne, Parkville VIC 3010,Australia email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

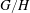We calculate equivariant elliptic cohomology of the partial flag variety $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}G/H$, where 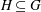$H\subseteq G$ are compact connected Lie groups of equal rank. We identify the 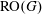${\rm RO}(G)$-graded coefficients 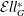${\mathcal{E}} ll_G^*$ as powers of Looijenga’s line bundle and prove that transfer along the map

$$\begin{equation*} \pi \,{:}\,G/H\longrightarrow {\rm pt} \end{equation*}$$
is calculated by the Weyl–Kac character formula. Treating ordinary cohomology, $K$-theory and elliptic cohomology in parallel, this paper organizes the theoretical framework for the elliptic Schubert calculus of [N. Ganter and A. Ram, Elliptic Schubert calculus, in preparation].

Keywords

elliptic cohomologyloop group characters

MSC classification

Secondary: 55N34: Elliptic cohomology 55N91: Equivariant homology and cohomology 19L47: Equivariant $K$-theory 22E67: Loop groups and related constructions, group-theoretic treatment
Type
Research Article
Information
Compositio Mathematica , Volume 150 , Issue 7 , July 2014 , pp. 1196 - 1234
Copyright
© The Author 2014 

References

Akyıldız, E. and Carrell, J. B., Zeros of holomorphic vector fields and the Gysin homomorphism, in Singularities, Part 1 (Arcata, CA, 1981), Proceedings of Symposia in Pure Mathematics, vol. 40 (American Mathematical Society, Providence, RI, 1983), 47–54; MR 713044 (85c:32020).Google Scholar
Ando, M., Power operations in elliptic cohomology and representations of loop groups, Trans. Amer. Math. Soc. 352 (2000), 5619–5666; MR 1637129 (2001b:55016).CrossRefGoogle Scholar
Ando, M., The sigma orientation for analytic circle-equivariant elliptic cohomology, Geom. Topol. 7 (2003), 91–153; MR 1988282 (2004d:55006).CrossRefGoogle Scholar
Ando, M., Hopkins, M. J. and Strickland, N. P., The sigma orientation is an H ∞map, Amer. J. Math. 126 (2004), 247–334; MR 2045503 (2005d:55009).Google Scholar
Atiyah, M. F. and Bott, R., A Lefschetz fixed point formula for elliptic complexes. II. Applications, Ann. of Math. (2) 88 (1968), 451–491; MR 0232406 (38 #731).Google Scholar
Atiyah, M. F. and Bott, R., The moment map and equivariant cohomology, Topology 23 (1984), 1–28; MR 721448 (85e:58041).Google Scholar
Atiyah, M. F. and Singer, I. M., The index of elliptic operators. I, Ann. of Math. (2) 87 (1968), 484–530; MR 0236950 (38 #5243).CrossRefGoogle Scholar
Atiyah, M. F. and Segal, G. B., Equivariant K-theory and completion, J. Differential Geom. 3 (1969), 1–18; MR 0259946 (41 #4575).Google Scholar
Baranovsky, V. and Ginzburg, V., Conjugacy classes in loop groups and G-bundles on elliptic curves, Int. Math. Res. Not. IMRN (1996), 733–751; MR 1413870 (97j:20044).CrossRefGoogle Scholar
Borel, A., Sur la cohomologie des espaces fibrés principaux et des espaces homogènes de groupes de Lie compacts, Ann. of Math. (2) 57 (1953), 115–207; MR 0051508 (14,490e).Google Scholar
Bott, R., On torsion in Lie groups, Proc. Natl. Acad. Sci. USA 40 (1954), 586–588; MR 0062750 (16,12a).Google Scholar
Bott, R. and Samelson, H., Applications of the theory of Morse to symmetric spaces, Amer. J. Math. 80 (1958), 964–1029; MR 0105694 (21 #4430).CrossRefGoogle Scholar
Boysal, A. and Kumar, S., Explicit determination of the Picard group of moduli spaces of semistable G-bundles on curves, Math. Ann. 332 (2005), 823–842; MR 2179779 (2006k:14061).Google Scholar
Bressler, P. and Evens, S., The Schubert calculus, braid relations, and generalized cohomology, Trans. Amer. Math. Soc. 317 (1990), 799–811; MR 968883 (90e:57074).Google Scholar
Bressler, P. and Evens, S., Schubert calculus in complex cobordism, Trans. Amer. Math. Soc. 331 (1992), 799–813; MR 1044959 (92h:57050).Google Scholar
Bröcker, T. and tom Dieck, T., Representations of compact Lie groups, Graduate Texts in Mathematics, vol. 98 (Springer, 1985).Google Scholar
Calmès, B., Petrov, V. and Zainoulline, K., Invariants, torsion indices and oriented cohomology of complete flags, Ann. Sci. Éc. Norm. Supér. (4) 46 (2013), 405–448; MR 3099981.Google Scholar
Dynkin, E. B., Semisimple subalgebras of semisimple Lie algebras, Mat. Sb. N.S. 30(72) (1952), 349–462; (3 plates) Translation: Amer. Math. Soc. Transl., Ser. II, 6 (1957), 111–244;MR 0047629 (13,904c).Google Scholar
Etingof, P. I. and Frenkel, I. B., Central extensions of current groups in two dimensions, Comm. Math. Phys. 165 (1994), 429–444; MR 1301619 (96e:22037).Google Scholar
Franke, J., On the construction of elliptic cohomology, Math. Nachr. 158 (1992), 43–65; MR 1235295 (94h:55007).Google Scholar
Friedman, R., Morgan, J. W. and Witten, E., Principal G-bundles over elliptic curves, Math. Res. Lett. 5 (1998), 97–118; MR 1618343 (99j:14037).Google Scholar
Ganter, N. and Ram, A., Elliptic Schubert calculus, in preparation.Google Scholar
Ganter, N. and Ram, A., Generalized Schubert calculus, Ramanujan Math. Soc., special volume in honor of Professor C. S. Seshadri on the occasion of his 80th birthday, to appear.Google Scholar
Gepner, D., Homotopy topoi and equivariant elliptic cohomology, PhD thesis, University of Illinois at Urbana-Champaign (2005).Google Scholar
Ginzburg, V., Kapranov, M. and Vasserot, E., Elliptic algebras and equivariant elliptic cohomology I, Technical report (1995), http://front.math.ucdavis.edu/9505.5152.Google Scholar
Goresky, M., Kottwitz, R. and MacPherson, R., Equivariant cohomology, Koszul duality, and the localization theorem, Invent. Math. 131 (1998), 25–83; MR 1489894 (99c:55009).Google Scholar
Grojnowski, I., Delocalised equivariant elliptic cohomology, in Elliptic cohomology, London Mathematical Society Lecture Note Series, vol. 342 (Cambridge University Press, Cambridge, 2007), 114–121; MR 2330510 (2008i:55006).Google Scholar
Harada, M., Henriques, A. and Holm, T. S., Computation of generalized equivariant cohomologies of Kac–Moody flag varieties, Adv. Math. 197 (2005), 198–221; MR 2166181 (2006h:53086).Google Scholar
Hilsum, M. and Skandalis, G., Morphismes K-orientés d’espaces de feuilles et fonctorialité en théorie de Kasparov (d’après une conjecture d’A. Connes), Ann. Sci. Éc. Norm. Supér. (4) 20 (1987), 325–390; MR 925720 (90a:58169).Google Scholar
Hirzebruch, F., Berger, T. and Jung, R., Manifolds and modular forms, Aspects of Mathematics, vol. E20 (Friedr. Vieweg & Sohn, Braunschweig, 1992), with appendices by N.-P. Skoruppa and by P. Baum; MR 1189136 (94d:57001).Google Scholar
Hoehn, G., Komplexe elliptische Geschlechter und $s^1$-quivariante Kobordismustheorie, Master’s thesis, Rheinische Friedrich Wilhelms Universität zu Bonn (1991).Google Scholar
Hornbostel, J. and Kiritchenko, V., Schubert calculus for algebraic cobordism, J. Reine Angew. Math. 656 (2011), 59–85; MR 2818856.Google Scholar
Kumar, S. and Narasimhan, M. S., Picard group of the moduli spaces of G-bundles, Math. Ann. 308 (1997), 155–173; MR 1446205 (98d:14014).CrossRefGoogle Scholar
Kumar, S., Narasimhan, M. S. and Ramanathan, A., Infinite Grassmannians and moduli spaces of G-bundles, Math. Ann. 300 (1994), 41–75; MR 1289830 (96e:14011).CrossRefGoogle Scholar
Landweber (ed), P. S., Elliptic curves and modular forms in algebraic topology, Lecture Notes in Mathematics, vol. 1326 (Springer, Berlin, 1988); MR 970278 (91a:57021).Google Scholar
Lerman, E., Orbifolds as stacks?, Enseign. Math. (2) 56 (2010), 315–363; MR 2778793 (2012c:18010).Google Scholar
Looijenga, E., Root systems and elliptic curves, Invent. Math. 38 (1976/77), 17–32; MR 0466134 (57 #6015).Google Scholar
Lurie, J., Survey article on elliptic cohomology, Preprint, http://www.math.harvard.edu/∼lurie/.Google Scholar
May, J. P., Equivariant homotopy and cohomology theory, CBMS Regional Conference Series in Mathematics, vol 91 (American Mathematical Society, Providence, RI, 1996), with contributions by M. Cole, G. Comezaña, S. Costenoble, A. D. Elmendorf, J. P. C. Greenlees, L. G. Lewis, Jr., R. J. Piacenza, G. Triantafillou, and S. Waner; MR 1413302 (97k:55016).Google Scholar
McLaughlin, D. A., Orientation and string structures on loop space, Pacific J. Math. 155 (1992), 143–156; MR 1174481 (93j:57015).Google Scholar
McLeod, J., The Kunneth formula in equivariant K-theory, in Algebraic topology, Waterloo, 1978 (Proc. Conf., Univ. Waterloo, Waterloo, Ont., 1978), Lecture Notes in Mathematics, vol. 741 (Springer, Berlin, 1979), 316–333; MR 557175 (80m:55007).CrossRefGoogle Scholar
Mimura, M. and Toda, H., Topology of Lie groups. I, II, Translations of Mathematical Monographs, vol. 91 (American Mathematical Society, Providence, RI, 1991), translated from the 1978 Japanese edition by the authors; MR 1122592 (92h:55001).Google Scholar
Pressley, A. and Segal, G., Loop groups, in Oxford Mathematical Monographs (The Clarendon Press, Oxford University Press, Oxford Science Publications, University Press, New York, 1986); MR 900587 (88i:22049).Google Scholar
Quillen, D., Elementary proofs of some results of cobordism theory using Steenrod operations, Adv. Math. 7 (1971), 29–56; MR 0290382 (44 #7566).Google Scholar
Roşu, I., Equivariant elliptic cohomology and rigidity, Amer. J. Math. 123 (2001), 647–677; MR 1844573 (2002e:55008).Google Scholar
Roşu, I., Equivariant K-theory and equivariant cohomology, Math. Z. 243 (2003), 423–448; with an appendix by A. Knutson and Roşu; MR 1970011 (2004f:19011).Google Scholar
Segal, G., Equivariant K-theory, Inst. Hautes Études Sci. Publ. Math. (1968), 129–151; MR 0234452 (38 #2769).CrossRefGoogle Scholar
Segal, G., The definition of conformal field theory, in Topology, geometry and quantum field theory, London Mathematical Society Lecture Note Series, vol. 308 (Cambridge University Press, Cambridge, 2004), 421–577; MR 2079383 (2005h:81334).Google Scholar
Serre, J.-P., Géométrie algébrique et géométrie analytique, Ann. Inst. Fourier, Grenoble 6 (1955–1956), 1–42; MR 0082175 (18,511a).CrossRefGoogle Scholar
Tymoczko, J., Divided difference operators for partial flag varieties, Preprint (2009),http://front.math.ucdavis.edu/0912.2545.Google Scholar