Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T00:59:29.116Z Has data issue: false hasContentIssue false
  • English
  • Français

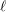$\ell$-independence for compatible systems of (mod $\ell$) representations

Part of: Discontinuous groups and automorphic forms (Co)homology theory Representation theory of groups

Published online by Cambridge University Press:  02 March 2015

Chun Yin Hui
Chun Yin Hui*
Affiliation:
University of Luxembourg, Mathematics Research Unit, 6 rue Richard Coudenhove-Kalergi, L-1359, Luxembourg email [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let 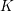$K$ be a number field. For any system of semisimple mod 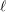$\ell$ Galois representations 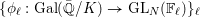$\{{\it\phi}_{\ell }:\text{Gal}(\bar{\mathbb{Q}}/K)\rightarrow \text{GL}_{N}(\mathbb{F}_{\ell })\}_{\ell }$ arising from étale cohomology (Definition 1), there exists a finite normal extension 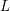$L$ of 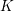$K$ such that if we denote 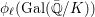${\it\phi}_{\ell }(\text{Gal}(\bar{\mathbb{Q}}/K))$ and 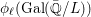${\it\phi}_{\ell }(\text{Gal}(\bar{\mathbb{Q}}/L))$ by 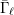$\bar{{\rm\Gamma}}_{\ell }$ and $\bar{{\it\gamma}}_{\ell }$, respectively, for all 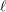$\ell$ and let 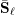$\bar{\mathbf{S}}_{\ell }$ be the 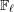$\mathbb{F}_{\ell }$-semisimple subgroup of 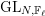$\text{GL}_{N,\mathbb{F}_{\ell }}$ associated to $\bar{{\it\gamma}}_{\ell }$ (or $\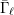bar{{\rm\Gamma}}_{\ell }$) by Nori’s theory [On subgroups of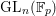$\text{GL}_{n}(\mathbb{F}_{p})$, Invent. Math. 88 (1987), 257–275] for sufficiently large 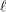$\ell$, then the following statements hold for all sufficiently large 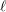$\ell$.

A(i) The formal character of 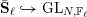$\bar{\mathbf{S}}_{\ell }{\hookrightarrow}\text{GL}_{N,\mathbb{F}_{\ell }}$ (Definition 1) is independent of 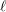$\ell$ and equal to the formal character of 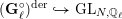$(\mathbf{G}_{\ell }^{\circ })^{\text{der}}{\hookrightarrow}\text{GL}_{N,\mathbb{Q}_{\ell }}$, where 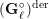$(\mathbf{G}_{\ell }^{\circ })^{\text{der}}$ is the derived group of the identity component of $\mathbf{G}_{\ell }$, the monodromy group of the corresponding semi-simplified $\ell$-adic Galois representation 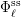${\rm\Phi}_{\ell }^{\text{ss}}$.

A(ii) The non-cyclic composition factors of 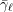$\bar{{\it\gamma}}_{\ell }$ and 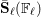$\bar{\mathbf{S}}_{\ell }(\mathbb{F}_{\ell })$ are identical. Therefore, the composition factors of 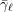$\bar{{\it\gamma}}_{\ell }$ are finite simple groups of Lie type of characteristic 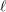$\ell$ and are cyclic groups.

B(i) The total 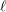$\ell$-rank 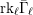$\text{rk}_{\ell }\bar{{\rm\Gamma}}_{\ell }$ of $\bar{{\rm\Gamma}}_{\ell }$ (Definition 14) is equal to the rank of 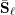$\bar{\mathbf{S}}_{\ell }$ and is therefore independent of 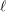$\ell$.

B(ii) The $A_{n}$-type 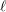$\ell$-rank 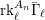$\text{rk}_{\ell }^{A_{n}}\bar{{\rm\Gamma}}_{\ell }$ of 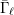$\bar{{\rm\Gamma}}_{\ell }$ (Definition 14) for 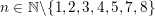$n\in \mathbb{N}\setminus \{1,2,3,4,5,7,8\}$ and the parity of 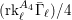$(\text{rk}_{\ell }^{A_{4}}\bar{{\rm\Gamma}}_{\ell })/4$ are independent of $\ell$.

Keywords

Galois representationsℓ-independencebig Galois imageétale cohomology

MSC classification

Primary: 11F80: Galois representations 14F20: Étale and other Grothendieck topologies and (co)homologies 20D05: Finite simple groups and their classification
Type
Research Article
Information
Compositio Mathematica , Volume 151 , Issue 7 , July 2015 , pp. 1215 - 1241
Copyright
© The Author 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Caruso, X., Conjecture de l’inertie modérée de Serre, Invent. Math. 171 (2008), 629–699.CrossRefGoogle Scholar
Cassels, J. W. S. and Fröhlich, A. (eds), Algebraic number theory: Proceedings of an instructional conference organized by the London Mathematical Society (University of Sussex, Brighton, September 1–17), second edition (Academic Press, London, 1965).Google Scholar
Curtis, C. W. and Reiner, I., Representation theory of finite groups and associative algebras, Wiley Classics Library, reprint of the 1962 original (John Wiley & Sons, New York, 1988).Google Scholar
de Jong, A. J., Smoothness, semi-stability and alterations, Publ. Math. Inst. Hautes Études Sci. 83 (1996), 51–93.CrossRefGoogle Scholar
Deligne, P., La conjecture de Weil I, Publ. Math. Inst. Hautes Études Sci. 43 (1974), 273–307.CrossRefGoogle Scholar
Dieudonné, J. and Grothendieck, A., Éléments de géométrie algébrique: IV. Étude locale des schémas et des morphismes de schémas, Seconde partie, Publ. Math. Inst. Hautes Études Sci. 24 (1965), 5–231.Google Scholar
Ellenberg, J., Hall, C. and Kowalski, E., Expander graphs, gonality, and variation of Galois representations, Duke Math. J. 161 (2012), 1233–1275.CrossRefGoogle Scholar
Faltings, G., Endlichkeitssätze für abelsche Varietäten über Zahlkörpern, Invent. Math. 73 (1983), 349–366.CrossRefGoogle Scholar
Gabber, O., Sur la torsion dans la cohomologie l-adique d’une variété, C. R. Acad. Sci. Paris 297 (1983), 179–182.Google Scholar
Hall, C., An open image theorem for a general class of abelian varieties, Bull. Lond. Math. Soc. 43 (2011), 703–711.CrossRefGoogle Scholar
Hartshorne, R., Algebraic geometry, Graduate Texts in Mathematics, vol. 52 (Springer, New York, 1977).CrossRefGoogle Scholar
Hui, C. Y., Monodromy of Galois representations and equal-rank subalgebra equivalence, Math. Res. Lett. 20 (2013), 705–728.CrossRefGoogle Scholar
Hui, C. Y. and Larsen, M., Type A images of Galois representations and maximality, Preprint (2014), arXiv:1305.1989.Google Scholar
Jordan, C., Mémoire sur les équations differentielles linéaires á intégrale algébrique, J. Reine Angew. Math. 84 (1878), 89–215.Google Scholar
Lang, S., Algebraic number theory, Graduate Texts in Mathematics, vol. 110, second edition (Springer, New York, 1994).CrossRefGoogle Scholar
Lang, S. and Wéil, A., Number of points of varieties in finite fields, Amer. J. Math. 76 (1954), 819–827.CrossRefGoogle Scholar
Milne, J. S., Lectures on étale cohomology (online course notes), http://www.jmilne.org/math/CourseNotes/LEC.pdf.Google Scholar
Neukirch, J., Algebraic number theory, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], vol. 322 (Springer, Berlin, 1999).CrossRefGoogle Scholar
Nori, M. V., On subgroups of GLn(Fp), Invent. Math. 88 (1987), 257–275.CrossRefGoogle Scholar
Serre, J.-P., Propriétés galoisiennes des points d’ordre fini des courbes elliptiques, Invent. Math. 15 (1972), 259–331.CrossRefGoogle Scholar
Serre, J.-P., Letter to K. A. Ribet, Jan. 1, 1981, reproduced in Oeuvres - Collected Papers IV, no. 133 (Springer, 2000).CrossRefGoogle Scholar
Serre, J.-P., Lettre á Marie-France Vignéras du 10/2/1986, reproduced in Oeuvres - Collected Papers IV, no. 137 (Springer, 2000).CrossRefGoogle Scholar
Serre, J.-P., Propriétés conjecturales des groupes de Galois motiviques et des représentations l-adiques, in Motives (Seattle, WA, 1991), Proceedings of Symposia in Pure Mathematics, vol. 55, Part 1 (American Mathematical Society, Providence, RI, 1994), 377–400.Google Scholar
Serre, J.-P., Abelian l-adic representation and elliptic curves, Research Notes in Mathematics, vol. 7, second edition (A. K. Peters, Wellesley, MA, 1998).Google Scholar
Springer, T. A., Linear algebraic groups, second edition (Birkhäuser, Boston, 2008).Google Scholar
Steinberg, R., Lectures on Chevalley groups (Yale University Press, New Haven, CT, 1968).Google Scholar
Steinberg, R., Endomorphisms of linear algebraic groups, Mem. Amer. Math. Soc. 80 (1968).Google Scholar