Article contents
Double affine Hecke algebras and generalized Jones polynomials
Published online by Cambridge University Press: 01 April 2016
Abstract
In this paper we propose and discuss implications of a general conjecture that there is a natural action of a rank 1 double affine Hecke algebra on the Kauffman bracket skein module of the complement of a knot 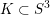 $K\subset S^{3}$. We prove this in a number of nontrivial cases, including all
$K\subset S^{3}$. We prove this in a number of nontrivial cases, including all 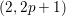 $(2,2p+1)$ torus knots, the figure eight knot, and all 2-bridge knots (when
$(2,2p+1)$ torus knots, the figure eight knot, and all 2-bridge knots (when  $q=\pm 1$). As the main application of the conjecture, we construct three-variable polynomial knot invariants that specialize to the classical colored Jones polynomials introduced by Reshetikhin and Turaev. We also deduce some new properties of the classical Jones polynomials and prove that these hold for all knots (independently of the conjecture). We furthermore conjecture that the skein module of the unknot is a submodule of the skein module of an arbitrary knot. We confirm this for the same example knots, and we show that this implies that the colored Jones polynomials of
$q=\pm 1$). As the main application of the conjecture, we construct three-variable polynomial knot invariants that specialize to the classical colored Jones polynomials introduced by Reshetikhin and Turaev. We also deduce some new properties of the classical Jones polynomials and prove that these hold for all knots (independently of the conjecture). We furthermore conjecture that the skein module of the unknot is a submodule of the skein module of an arbitrary knot. We confirm this for the same example knots, and we show that this implies that the colored Jones polynomials of 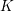 $K$ satisfy an inhomogeneous recursion relation.
$K$ satisfy an inhomogeneous recursion relation.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Authors 2016
References
- 13
- Cited by


