Hostname: page-component-cc8bf7c57-ksm4s Total loading time: 0 Render date: 2024-12-11T22:09:48.341Z Has data issue: false hasContentIssue false
  • English
  • Français

Derived categories of 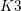$K3$ surfaces, O’Grady’s filtration, and zero-cycles on holomorphic symplectic varieties

Part of: Surfaces and higher-dimensional varieties Cycles and subschemes (Co)homology theory

Published online by Cambridge University Press:  26 November 2019

Junliang Shen ,
Qizheng Yin  and
Xiaolei Zhao
Junliang Shen
Affiliation:
Massachusetts Institute of Technology, Department of Mathematics, Simons Building, 77 Massachusetts Avenue, Cambridge, MA 02139, USA email [email protected]
Qizheng Yin
Affiliation:
Peking University, Beijing International Center for Mathematical Research, Jingchunyuan Courtyard #78, 5 Yiheyuan Road, Haidian District, Beijing 100871, China email [email protected]
Xiaolei Zhao
Affiliation:
University of California, Santa Barbara, Department of Mathematics, South Hall, Santa Barbara, CA 93106, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Moduli spaces of stable objects in the derived category of a $K3$ surface provide a large class of holomorphic symplectic varieties. In this paper, we study the interplay between Chern classes of stable objects and zero-cycles on holomorphic symplectic varieties which arise as moduli spaces. First, we show that the second Chern class of any object in the derived category lies in a suitable piece of O’Grady’s filtration on the $\text{CH}_{0}$-group of the 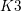$K3$ surface. This solves a conjecture of O’Grady and improves on previous results of Huybrechts, O’Grady, and Voisin. Second, we propose a candidate for the Beauville–Voisin filtration on the $\text{CH}_{0}$-group of the moduli space of stable objects. We discuss its connection with Voisin’s recent proposal via constant cycle subvarieties, and prove a conjecture of hers on the existence of special algebraically coisotropic subvarieties for the moduli space.

Keywords

K3 surfacesderived categoriesChow groupsmoduli spaces of sheaveshyper-Kähler varieties

MSC classification

Primary: 14J28: $K3$ surfaces and Enriques surfaces 14F05: Sheaves, derived categories of sheaves and related constructions 14C15: (Equivariant) Chow groups and rings; motives
Type
Research Article
Information
Compositio Mathematica , Volume 156 , Issue 1 , January 2020 , pp. 179 - 197
Copyright
© The Authors 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bayer, A. and Bridgeland, T., Derived automorphism groups of K3 surfaces of Picard rank 1 , Duke Math. J. 166 (2017), 75–124.Google Scholar
Bayer, A. and Macrì, E., Projectivity and birational geometry of Bridgeland moduli spaces , J. Amer. Math. Soc. 27 (2014), 707–752.Google Scholar
Bayer, A. and Macrì, E., MMP for moduli of sheaves on K3s via wall-crossing: nef and movable cones, Lagrangian fibrations , Invent. Math. 198 (2014), 505–590.Google Scholar
Beauville, A., Variétés kählériennes dont la première classe de Chern est nulle , J. Differential Geom. 18 (1983), 755–782 (1984).Google Scholar
Beauville, A., On the splitting of the Bloch–Beilinson filtration , in Algebraic cycles and motives, Vol. 2, London Mathematical Society Lecture Note Series, vol. 344 (Cambridge University Press, Cambridge, 2007), 38–53.Google Scholar
Beauville, A. and Voisin, C., On the Chow ring of a K3 surface , J. Algebraic Geom. 13 (2004), 417–426.Google Scholar
Bridgeland, T., Stability conditions on triangulated categories , Ann. of Math. (2) 166 (2007), 317–345.Google Scholar
Bridgeland, T., Stability conditions on K3 surfaces , Duke Math. J. 141 (2008), 241–291.Google Scholar
Huybrechts, D., Birational symplectic manifolds and their deformations , J. Differential Geom. 45 (1997), 488–513.Google Scholar
Huybrechts, D., Compact hyper-Kähler manifolds: basic results , Invent. Math. 135 (1999), 63–113.Google Scholar
Huybrechts, D., Chow groups of K3 surfaces and spherical objects , J. Eur. Math. Soc. (JEMS) 12 (2010), 1533–1551.Google Scholar
Huybrechts, D., Curves and cycles on K3 surfaces , Algebr. Geom. 1 (2014), 69–106.Google Scholar
Huybrechts, D., Motives of isogenous K3 surfaces , Comment. Math. Helv. 94 (2019), 445–458.Google Scholar
Kuznetsov, A., Derived categories of cubic fourfolds , in Cohomological and geometric approaches to rationality problems, Progress in Mathematics, vol. 282 (Birkhäuser, Boston, MA, 2010), 219–243.Google Scholar
Maclean, C., Chow groups of surfaces with h 2, 0⩽1 , C. R. Math. Acad. Sci. Paris 338 (2004), 55–58.Google Scholar
Marian, A. and Zhao, X., On the group of zero-cycles of holomorphic symplectic varieties, Preprint (2017), arXiv:1711.10045v2.Google Scholar
Mukai, S., Symplectic structure of the moduli space of sheaves on an Abelian or K3 surface , Invent. Math. 77 (1984), 101–116.Google Scholar
Mukai, S., On the moduli space of bundles on K3 surfaces. I , in Vector bundles on algebraic varieties, Bombay, 1984, Tata Institute of Fundamental Research Studies in Mathematics, vol. 11 (Tata Institute of Fundamental Research, Bombay, 1987), 341–413.Google Scholar
O’Grady, K. G., The weight-two Hodge structure of moduli spaces of sheaves on a K3 surface , J. Algebraic Geom. 6 (1997), 599–644.Google Scholar
O’Grady, K. G., Moduli of sheaves and the Chow group of K3 surfaces , J. Math. Pures Appl. (9) 100 (2013), 701–718.Google Scholar
Rieß, U., On the Chow ring of birational irreducible symplectic varieties , Manuscripta Math. 145 (2014), 473–501.Google Scholar
Shen, J. and Yin, Q., $K3$ categories, one-cycles on cubic fourfolds, and the Beauville–Voisin filtration, J. Inst. Math. Jussieu, doi:10.1017/S147474801800049X.Google Scholar
Voisin, C., On the Chow ring of certain algebraic hyper-Kähler manifolds , Pure Appl. Math. Q. 4 (2008), 613–649.Google Scholar
Voisin, C., Rational equivalence of 0-cycles on K3 surfaces and conjectures of Huybrechts and O’Grady , in Recent advances in algebraic geometry, London Mathematical Society Lecture Note Series, vol. 417 (Cambridge University Press, Cambridge, 2015), 422–436.Google Scholar
Voisin, C., Remarks and questions on coisotropic subvarieties and 0-cycles of hyper-Kähler varieties , in K3 surfaces and their moduli, Progress in Mathematics, vol. 315 (Birkhäuser/Springer, Cham, 2016), 365–399.Google Scholar
Yoshioka, K., Moduli spaces of stable sheaves on Abelian surfaces , Math. Ann. 321 (2001), 817–884.Google Scholar