Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-18T08:57:00.936Z Has data issue: false hasContentIssue false
  • English
  • Français

Corrigendum: ‘On certain algebraic curves related to polynomial maps, Compositio Math. 103 (1996), 319–350’

Part of: Arithmetic and non-Archimedean dynamical systems

Published online by Cambridge University Press:  18 June 2010

Patrick Morton
Patrick Morton*
Affiliation:
Department of Mathematical Sciences, Indiana University–Purdue University at Indianapolis, 402 N. Blackford St, LD 270, Indianapolis, Indiana 46202, USA (email: [email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An argument is given to fill a gap in a proof in the author’s article On certain algebraic curves related to polynomial maps, Compositio Math. 103 (1996), 319–350, that the polynomial Φn(x,c), whose roots are the periodic points of period n of a certain polynomial map x→f(x,c), is absolutely irreducible over the finite field of p elements, provided that f(x,1) has distinct roots and that the multipliers of the orbits of period n are also distinct over 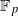. Assuming that Φn(x,c) is reducible in characteristic p, we show that Hensel’s lemma and Laurent series expansions of the roots can be used to obtain a factorization of Φn(x,c) in characteristic 0, contradicting the absolute irreducibility of this polynomial over the rational field.

Keywords

dynatomic polynomialabsolute irreducibilityHensel’s lemmaLaurent series expansions

MSC classification

Secondary: 37P05: Polynomial and rational maps 37P25: Finite ground fields
Type
Corrigenda
Information
Compositio Mathematica , Volume 147 , Issue 1 , January 2011 , pp. 332 - 334
Copyright
Copyright © Foundation Compositio Mathematica 2010

References

[1]Hasse, H., Zahlentheorie (Akademie Verlag, Berlin, 1969).CrossRefGoogle Scholar
[2]Morton, P., On certain algebraic curves related to polynomial maps, Compositio Math. 103 (1996), 319–350.Google Scholar
[3]Morton, P., Galois groups of periodic points, J. Algebra 201 (1998), 401–428.CrossRefGoogle Scholar
[4]Silverman, J. H., The arithmetic of dynamical systems, Springer Graduate Texts in Mathematics, vol. 241 (Springer, Berlin, 2007).CrossRefGoogle Scholar