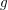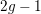Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yasui, Kouichi
2016.
Maximal Thurston–Bennequin number and reducible Legendrian surgery.
Compositio Mathematica,
Vol. 152,
Issue. 9,
p.
1899.
Cochran, Tim D.
and
Ray, Arunima
2016.
Shake slice and shake concordant knots.
Journal of Topology,
Vol. 9,
Issue. 3,
p.
861.
Leverson, Caitlin
2017.
Augmentations and rulings of Legendrian links in #k(S1× S2).
Pacific Journal of Mathematics,
Vol. 288,
Issue. 2,
p.
381.
Baldwin, John A
and
Sivek, Steven
2021.
On the equivalence of contact invariants in sutured Floer homology theories.
Geometry & Topology,
Vol. 25,
Issue. 3,
p.
1087.
McCullough, Andrew
2023.
Legendrian large cables and new phenomenon for nonuniformly thick knots.
Algebraic & Geometric Topology,
Vol. 23,
Issue. 6,
p.
2561.