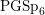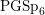Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gan, Wee Teck
and
Savin, Gordan
2023.
The Local Langlands Conjecture for.
Forum of Mathematics, Pi,
Vol. 11,
Issue. ,
Cauchi, Antonio
and
Terradillos, Armando Gutierrez
2024.
A two variable Rankin–Selberg integral for $${\textrm{GU}}(2,2)$$ and the degree 5 L-function of $${\textrm{GSp}}_4$$.
Mathematische Zeitschrift,
Vol. 308,
Issue. 2,
Gan, Wee Teck
and
Wang, Bryan Peng Jun
2025.
Generalised Whittaker models as instances of relative Langlands duality.
Advances in Mathematics,
Vol. 463,
Issue. ,
p.
110129.
Cauchi, Antonio
Lemma, Francesco
and
Rodrigues Jacinto, Joaquín
2025.
Algebraic cycles and functorial lifts from G2 to
PGSp6.
Algebra & Number Theory,
Vol. 19,
Issue. 3,
p.
551.
Pollack, Aaron
2025.
Exceptional Siegel Weil theorems for compact $${{\,\textrm{Spin}\,}}_8$$.
Mathematische Annalen,
Vol. 391,
Issue. 3,
p.
4271.