Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T05:17:02.919Z Has data issue: false hasContentIssue false
  • English
  • Français

Adelic descent theory

Part of: Families, fibrations (Co)homology theory

Published online by Cambridge University Press:  31 May 2017

Michael Groechenig
Michael Groechenig*
Affiliation:
Department of Mathematics and Computer Science, Freie Universität Berlin, D-14195 Berlin, Germany email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A result of André Weil allows one to describe rank $n$ vector bundles on a smooth complete algebraic curve up to isomorphism via a double quotient of the set 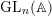$\text{GL}_{n}(\mathbb{A})$ of regular matrices over the ring of adèles (over algebraically closed fields, this result is also known to extend to $G$-torsors for a reductive algebraic group $G$). In the present paper we develop analogous adelic descriptions for vector and principal bundles on arbitrary Noetherian schemes, by proving an adelic descent theorem for perfect complexes. We show that for Beilinson’s co-simplicial ring of adèles $\mathbb{A}_{X}^{\bullet }$, we have an equivalence 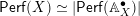$\mathsf{Perf}(X)\simeq |\mathsf{Perf}(\mathbb{A}_{X}^{\bullet })|$ between perfect complexes on $X$ and cartesian perfect complexes for 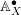$\mathbb{A}_{X}^{\bullet }$. Using the Tannakian formalism for symmetric monoidal 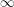$\infty$-categories, we conclude that a Noetherian scheme can be reconstructed from the co-simplicial ring of adèles. We view this statement as a scheme-theoretic analogue of Gelfand–Naimark’s reconstruction theorem for locally compact topological spaces from their ring of continuous functions. Several results for categories of perfect complexes over (a strong form of) flasque sheaves of algebras are established, which might be of independent interest.

Keywords

adèlesvector bundlesperfect complexesmoduli stacks

MSC classification

Primary: 14D23: Stacks and moduli problems 14F05: Sheaves, derived categories of sheaves and related constructions
Type
Research Article
Information
Compositio Mathematica , Volume 153 , Issue 8 , August 2017 , pp. 1706 - 1746
Copyright
© The Author 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Beauville, A. and Laszlo, Y., Conformal blocks and generalized theta functions , Comm. Math. Phys. 164 (1994), 385–419; MR 1289330 (95k:14011).CrossRefGoogle Scholar
Beauville, A. and Laszlo, Y., Un lemme de descente , C. R. Acad. Sci. Paris Sér. I Math. 320 (1995), 335–340; MR 1320381 (96a:14049).Google Scholar
Beilinson, A., Residues and adèles , Funktsional. Anal. i Prilozhen. 14 (1980), 44–45; MR 565095 (81f:14010).CrossRefGoogle Scholar
Ben-Bassat, O. and Temkin, M., Berkovich spaces and tubular descent , Adv. Math. 234 (2013), 217–238; MR 3003930.CrossRefGoogle Scholar
Bhatt, B., Algebraization and Tannaka duality , Camb. J. Math. 4 (2016), 403–461; MR 3572635.Google Scholar
Bhatt, B. and Halpern-Leistner, D., Tannaka duality revisited, Preprint (2015), arXiv:1507.01925.Google Scholar
Bhatt, B. and Scholze, P., The pro-étale topology for schemes , Astérisque 369 (2015), 99–201; MR 3379634.Google Scholar
Bondal, A. and Van den Bergh, M., Generators and representability of functors in commutative and noncommutative geometry , Mosc. Math. J. 3 (2003), 1–36.CrossRefGoogle Scholar
Bousfield, A. K., Cosimplicial resolutions and homotopy spectral sequences in model categories , Geom. Topol. 7 (2003), 1001–1053.Google Scholar
Braunling, O., Groechenig, M. and Wolfson, J., A generalized Contou–Carrère symbol and its reciprocity laws in higher dimensions, Preprint (2014), arXiv:1410.3451.Google Scholar
Bühler, T., Exact categories , Expo. Math. 28 (2010), 1–69; MR 2606234 (2011e:18020).Google Scholar
Efimov, A. I., Formal completion of a category along a subcategory, Preprint (2010), arXiv:1006.4721.Google Scholar
Elmendorf, A. D., Kriz, I., Mandell, M. A. and May, J. P., Rings, modules, and algebras in stable homotopy theory, Mathematical Surveys and Monographs, vol. 47 (American Mathematical Society, Providence, RI, 2007).CrossRefGoogle Scholar
Frenkel, E., Lectures on the Langlands program and conformal field theory , in Frontiers in number theory, physics, and geometry II (Springer, Berlin, 2007), 387–533.Google Scholar
Garland, H. and Patnaik, M., Geometry of loop Eisenstein series, Preprint, available athttps://sites.ualberta.ca/∼patnaik/gle.pdf.Google Scholar
Gelfand, I. M. and Naimark, M. A., On the embedding of normed linear rings into the ring of operators in Hilbert space , Mat. Sb. 12 (1943), 197–213.Google Scholar
Grothendieck, A. and Illusie, L., Séminaire de Géométrie Algébrique du Bois Marie - 1966–67 - Théorie des intersections et théorème de Riemann–Roch (SGA 6), Lecture Notes in Mathematics, vol. 225 (Springer, Berlin, 1971).Google Scholar
Hall, J. and Rydh, D., Algebraic groups and compact generation of their derived categories of representations , Indiana Univ. Math. J. 64 (2015), 1903–1923; MR 3436239.CrossRefGoogle Scholar
Hennion, B., Porta, M. and Vezzosi, G., Formal gluing for non-linear flags, Preprint (2016), arXiv:1607.04503.Google Scholar
Huber, A., On the Parshin–Beĭlinson adèles for schemes , Abh. Math. Semin. Univ. Hambg 61 (1991), 249–273; MR 1138291 (92k:14024).Google Scholar
Keller, B., Derived categories and their uses , Handb. Algebr. 1 (1996), 671–701.Google Scholar
Lieblich, M., Moduli of complexes on a proper morphism , J. Algebraic Geom. 15 (2006), 175–206; MR 2177199.CrossRefGoogle Scholar
Lurie, J., Higher algebra, Preprint, available at http://www.math.harvard.edu/∼lurie.Google Scholar
Lurie, J., Higher topos theory, Preprint (2007), available at http://www-math.mit.edu/∼lurie/papers/highertopoi.pdf.Google Scholar
Lurie, J., Quasi-coherent sheaves and Tannaka duality theorems, Preprint (2011), available at http://www.math.harvard.edu/lurie.Google Scholar
Meyer, J.-P., Cosimplicial homotopies , Proc. Amer. Math. Soc. 108 (1990), 9–17.Google Scholar
Morrow, M., An introduction to higher dimensional local fields and adèles, Preprint (2012), arXiv:1204.0586.Google Scholar
Neeman, A., The grothendieck duality theorem via Bousfield’s techniques and Brown representability , J. Amer. Math. Soc. 9 (1996), 205–236.Google Scholar
Parshin, A. N., Chern classes, adèles and L-functions , J. Reine Angew. Math. 341 (1983), 174–192; MR 697316 (85c:14015).Google Scholar
Stacks Project Authors, Stacks project,http://math.columbia.edu/algebraic_geometry/stacks-git.Google Scholar
Thomason, R. W. and Trobaugh, T., Higher algebraic K-theory of schemes and of derived categories , in The Grothendieck Festschrift, Vol. III, Progress in Mathematics, vol. 88 (Birkhäuser, Boston, MA, 1990), 247–435; MR 1106918 (92f:19001).Google Scholar
Toën, B. and Vezzosi, G., Homotopical algebraic geometry. II. Geometric stacks and applications , Mem. Amer. Math. Soc. 193 (2008), MR 2394633 (2009h:14004).Google Scholar
Weil, A., Généralisation des fonctions abéliennes , J. Math. Pures Appl. (9) 17 (1938), 47–87 (French).Google Scholar
Weil, A., Zur algebraischen theorie der algebraischen Funktionen , J. Reine Angew. Math. 179 (1938), 129–133.Google Scholar
Witten, E., Quantum field theory, Grassmannians, and algebraic curves , Comm. Math. Phys. 113 (1988), 529–600.CrossRefGoogle Scholar