No CrossRef data available.
Article contents
Sets of special subvarieties of bounded degree
Published online by Cambridge University Press: 14 March 2023
Abstract
Let 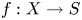 $f : X \to S$ be a family of smooth projective algebraic varieties over a smooth connected quasi-projective base
$f : X \to S$ be a family of smooth projective algebraic varieties over a smooth connected quasi-projective base  $S$, and let
$S$, and let 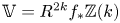 $\mathbb {V} = R^{2k} f_{*} \mathbb {Z}(k)$ be the integral variation of Hodge structure coming from degree
$\mathbb {V} = R^{2k} f_{*} \mathbb {Z}(k)$ be the integral variation of Hodge structure coming from degree 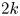 $2k$ cohomology it induces. Associated to
$2k$ cohomology it induces. Associated to 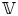 $\mathbb {V}$ one has the so-called Hodge locus
$\mathbb {V}$ one has the so-called Hodge locus 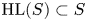 $\textrm {HL}(S) \subset S$, which is a countable union of ‘special’ algebraic subvarieties of
$\textrm {HL}(S) \subset S$, which is a countable union of ‘special’ algebraic subvarieties of 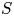 $S$ parametrizing those fibres of
$S$ parametrizing those fibres of  $\mathbb {V}$ possessing extra Hodge tensors (and so, conjecturally, those fibres of
$\mathbb {V}$ possessing extra Hodge tensors (and so, conjecturally, those fibres of 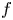 $f$ possessing extra algebraic cycles). The special subvarieties belong to a larger class of so-called weakly special subvarieties, which are subvarieties of
$f$ possessing extra algebraic cycles). The special subvarieties belong to a larger class of so-called weakly special subvarieties, which are subvarieties of 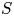 $S$ maximal for their algebraic monodromy groups. For each positive integer
$S$ maximal for their algebraic monodromy groups. For each positive integer  $d$, we give an algorithm to compute the set of all weakly special subvarieties
$d$, we give an algorithm to compute the set of all weakly special subvarieties 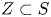 $Z \subset S$ of degree at most
$Z \subset S$ of degree at most 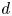 $d$ (with the degree taken relative to a choice of projective compactification
$d$ (with the degree taken relative to a choice of projective compactification 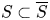 $S \subset \overline {S}$ and very ample line bundle
$S \subset \overline {S}$ and very ample line bundle 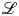 $\mathcal {L}$ on
$\mathcal {L}$ on 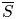 $\overline {S}$). As a corollary of our algorithm we prove conjectures of Daw–Ren and Daw–Javanpeykar–Kühne on the finiteness of sets of special and weakly special subvarieties of bounded degree.
$\overline {S}$). As a corollary of our algorithm we prove conjectures of Daw–Ren and Daw–Javanpeykar–Kühne on the finiteness of sets of special and weakly special subvarieties of bounded degree.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2023 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence



