Article contents
Relative hyperbolicity of free-by-cyclic extensions
Published online by Cambridge University Press: 30 January 2023
Abstract
Given a finitely generated free group 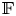 $ {\mathbb {F} }$ of
$ {\mathbb {F} }$ of 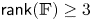 $\mathsf {rank}( {\mathbb {F} } )\geq 3$, we show that the mapping torus of
$\mathsf {rank}( {\mathbb {F} } )\geq 3$, we show that the mapping torus of  $\phi$ is (strongly) relatively hyperbolic if
$\phi$ is (strongly) relatively hyperbolic if 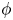 $\phi$ is exponentially growing. As a corollary of our work, we give a new proof of Brinkmann's theorem which proves that the mapping torus of an atoroidal outer automorphism is hyperbolic. We also give a new proof of the Bridson–Groves theorem that the mapping torus of a free group automorphism satisfies the quadratic isoperimetric inequality. Our work also solves a problem posed by Minasyan and Osin: the mapping torus of an outer automorphism is not virtually acylindrically hyperbolic if and only if
$\phi$ is exponentially growing. As a corollary of our work, we give a new proof of Brinkmann's theorem which proves that the mapping torus of an atoroidal outer automorphism is hyperbolic. We also give a new proof of the Bridson–Groves theorem that the mapping torus of a free group automorphism satisfies the quadratic isoperimetric inequality. Our work also solves a problem posed by Minasyan and Osin: the mapping torus of an outer automorphism is not virtually acylindrically hyperbolic if and only if 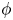 $\phi$ has finite order.
$\phi$ has finite order.
Keywords
MSC classification
- Type
- Research Article
- Information
- Copyright
- © 2023 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence
Footnotes
The author was supported by a faculty research grant from Ashoka University during this project.
References
- 2
- Cited by



