Article contents
A motivic version of the theorem of Fontaine and Wintenberger
Published online by Cambridge University Press: 23 November 2018
Abstract
We establish a tilting equivalence for rational, homotopy-invariant cohomology theories defined over non-archimedean analytic varieties. More precisely, we prove an equivalence between the categories of motives of rigid analytic varieties over a perfectoid field  $K$ of mixed characteristic and over the associated (tilted) perfectoid field
$K$ of mixed characteristic and over the associated (tilted) perfectoid field  $K^{\flat }$ of equal characteristic. This can be considered as a motivic generalization of a theorem of Fontaine and Wintenberger, claiming that the Galois groups of
$K^{\flat }$ of equal characteristic. This can be considered as a motivic generalization of a theorem of Fontaine and Wintenberger, claiming that the Galois groups of 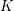 $K$ and
$K$ and 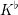 $K^{\flat }$ are isomorphic.
$K^{\flat }$ are isomorphic.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author 2018
References
- 5
- Cited by


