Article contents
The 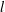 $l$-parity conjecture for abelian varieties over function fields of characteristic
$l$-parity conjecture for abelian varieties over function fields of characteristic 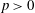 $p>0$
$p>0$
Published online by Cambridge University Press: 10 March 2014
Abstract
Let  $A/K$ be an abelian variety over a function field of characteristic
$A/K$ be an abelian variety over a function field of characteristic  $p>0$ and let
$p>0$ and let 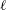 $\ell $ be a prime number (
$\ell $ be a prime number ( $\ell =p$ allowed). We prove the following: the parity of the corank
$\ell =p$ allowed). We prove the following: the parity of the corank 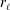 $r_\ell $ of the
$r_\ell $ of the  $\ell $-discrete Selmer group of
$\ell $-discrete Selmer group of 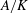 $A/K$ coincides with the parity of the order at
$A/K$ coincides with the parity of the order at 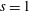 $s=1$ of the Hasse–Weil
$s=1$ of the Hasse–Weil 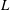 $L$-function of
$L$-function of  $A/K$. We also prove the analogous parity result for pure
$A/K$. We also prove the analogous parity result for pure 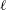 $\ell $-adic sheaves endowed with a nice pairing and in particular for the congruence Zeta function of a projective smooth variety over a finite field. Finally, we prove that the full Birch and Swinnerton-Dyer conjecture is equivalent to the Artin–Tate conjecture.
$\ell $-adic sheaves endowed with a nice pairing and in particular for the congruence Zeta function of a projective smooth variety over a finite field. Finally, we prove that the full Birch and Swinnerton-Dyer conjecture is equivalent to the Artin–Tate conjecture.
- Type
- Research Article
- Information
- Copyright
- © The Author(s) 2014
References
- 3
- Cited by


