Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-25T06:03:06.244Z Has data issue: false hasContentIssue false
  • English
  • Français

Le principe de Hasse pour les espaces homogènes : réduction au cas des stabilisateurs finis

Part of: Forms and linear algebraic groups Algebraic groups

Published online by Cambridge University Press:  04 July 2019

Cyril Demarche  and
Giancarlo Lucchini Arteche
Cyril Demarche
Affiliation:
Sorbonne Université, Institut de Mathématiques de Jussieu – Paris Rive Gauche, UMR 7586, CNRS, Université Paris Diderot, 75005 Paris, France Département de mathématiques et applications, École normale supérieure, CNRS, PSL Research University, 45 rue d’Ulm, 75005 Paris, France email [email protected]
Giancarlo Lucchini Arteche
Affiliation:
Departamento de Matemáticas, Facultad de Ciencias, Universidad de Chile, Las Palmeras 3425, Ñuñoa, Santiago, Chile email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Nous montrons, pour une grande famille de propriétés des espaces homogènes, qu’une telle propriété vaut pour tout espace homogène d’un groupe linéaire connexe dès qu’elle vaut pour les espaces homogènes de 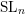$\text{SL}_{n}$ à stabilisateur fini. Nous réduisons notamment à ce cas particulier la vérification d’une importante conjecture de Colliot-Thélène sur l’obstruction de Brauer–Manin au principe de Hasse et à l’approximation faible. Des travaux récents de Harpaz et Wittenberg montrent que le résultat principal s’applique également à la conjecture analogue (dite conjecture (E)) pour les zéro-cycles.

For a large family of properties of homogeneous spaces, we prove that such a property holds for all homogeneous spaces of connected linear algebraic groups as soon as it holds for homogeneous spaces of $\text{SL}_{n}$ with finite stabilisers. As an example, we reduce to this particular case an important conjecture by Colliot-Thélène about the Brauer–Manin obstruction to the Hasse principle and to weak approximation. A recent work by Harpaz and Wittenberg proves that the main result also applies to the analogous conjecture (known as conjecture (E)) for zero-cycles on homogeneous spaces.

Keywords

espaces homogènesgerbesprincipe de Hasseobstruction de Brauer–Manin

MSC classification

Primary: 11E72: Galois cohomology of linear algebraic groups 14L30: Group actions on varieties or schemes (quotients)
Type
Research Article
Information
Compositio Mathematica , Volume 155 , Issue 8 , August 2019 , pp. 1568 - 1593
Copyright
© The Authors 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Borovoi, M., Abelianization of the second nonabelian Galois cohomology , Duke Math. J. 72 (1993), 217–239.Google Scholar
Borovoi, M., The Brauer–Manin obstructions for homogeneous spaces with connected or abelian stabilizer , J. Reine Angew. Math. 473 (1996), 181–194.Google Scholar
Borovoi, M., Colliot-Thélène, J.-L. and Skorobogatov, A., The elementary obstruction and homogeneous spaces , Duke Math. J. 141 (2008), 321–364.Google Scholar
Bosch, S., Lütkebohmert, W. and Raynaud, M., Néron models, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), vol. 21 (Springer, Berlin, 1990).Google Scholar
Breen, L., On the classification of 2-gerbes and 2-stacks, Astérisque, vol. 225 (Société Mathématique de France, Paris, 1994).Google Scholar
Colliot-Thélène, J.-L., Points rationnels sur les fibrations , in Higher dimensional varieties and rational points (Budapest, 2001), Bolyai Society Mathematical Studies, vol. 12 (Springer, Berlin, 2003), 171–221.Google Scholar
Colliot-Thélène, J.-L. and Sansuc, J.-J., The rationality problem for fields of invariants under linear algebraic groups (with special regards to the Brauer group) , in Algebraic groups and homogeneous spaces, Tata Institute of Fundamental Research Studies in Mathematics (Tata Institute of Fundamental Research, Mumbai, 2007), 113–186.Google Scholar
Demarche, C., Groupe de Brauer non ramifié d’espaces homogènes à stabilisateurs finis , Math. Ann. 346 (2010), 949–968.Google Scholar
Demazure, M. and Gabriel, P., Groupes algébriques. Tome I: Géométrie algébrique, généralités, groupes commutatifs (Masson & Cie, Paris; North-Holland, Amsterdam, 1970).Google Scholar
Flicker, Y. Z., Scheiderer, C. and Sujatha, R., Grothendieck’s theorem on non-abelian H 2 and local–global principles , J. Amer. Math. Soc. 11 (1998), 731–750.Google Scholar
Florence, M., Points rationnels sur les espaces homogènes et leurs compactifications , Transform. Groups 11 (2006), 161–176.Google Scholar
Giraud, J., Méthode de la descente , Bull. Soc. Math. France Mém. 2 (1964), III-1–VIII-150.Google Scholar
Giraud, J., Cohomologie non abélienne, Grundlehren der mathematischen Wissenschaften, vol. 179 (Springer, Berlin–New York, 1971).Google Scholar
Harpaz, Y. and Wittenberg, O., Zéro-cycles sur les espaces homogènes et problème de Galois inverse, Preprint (2018), arXiv:1802.09605.Google Scholar
Lucchini Arteche, G., Approximation faible et principe de Hasse pour des espaces homogènes à stabilisateur fini résoluble , Math. Ann. 360 (2014), 1021–1039.Google Scholar
Lucchini Arteche, G., Weak approximation for homogeneous spaces: reduction to the case with finite stabilizer , Math. Res. Lett. 24 (2017), 1–19.Google Scholar
Sansuc, J.-J., Groupe de Brauer et arithmétique des groupes algébriques linéaires sur un corps de nombres , J. Reine Angew. Math. 327 (1981), 12–80.Google Scholar
Skorobogatov, A., Torsors and rational points, Cambridge Tracts in Mathematics, vol. 144 (Cambridge University Press, Cambridge, 2001).Google Scholar
Springer, T. A., Nonabelian H 2 in Galois cohomology , in Algebraic groups and discontinuous subgroups (Proc. Sympos. Pure Math., Boulder, CO, 1965) (American Mathematical Society, Providence, RI, 1966), 164–182.Google Scholar
The Stacks Project Authors, Stacks project(2018), available at https://stacks.math.columbia.edu.Google Scholar
Wittenberg, O., Zéro-cycles sur les fibrations au-dessus d’une courbe de genre quelconque , Duke Math. J. 161 (2012), 2113–2166.Google Scholar