Article contents
Galois level and congruence ideal for 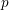 $p$-adic families of finite slope Siegel modular forms
$p$-adic families of finite slope Siegel modular forms
Published online by Cambridge University Press: 27 March 2019
Abstract
We consider families of Siegel eigenforms of genus 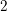 $2$ and finite slope, defined as local pieces of an eigenvariety and equipped with a suitable integral structure. Under some assumptions on the residual image, we show that the image of the Galois representation associated with a family is big, in the sense that a Lie algebra attached to it contains a congruence subalgebra of non-zero level. We call the Galois level of the family the largest such level. We show that it is trivial when the residual representation has full image. When the residual representation is a symmetric cube, the zero locus defined by the Galois level of the family admits an automorphic description: it is the locus of points that arise from overconvergent eigenforms for
$2$ and finite slope, defined as local pieces of an eigenvariety and equipped with a suitable integral structure. Under some assumptions on the residual image, we show that the image of the Galois representation associated with a family is big, in the sense that a Lie algebra attached to it contains a congruence subalgebra of non-zero level. We call the Galois level of the family the largest such level. We show that it is trivial when the residual representation has full image. When the residual representation is a symmetric cube, the zero locus defined by the Galois level of the family admits an automorphic description: it is the locus of points that arise from overconvergent eigenforms for 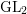 $\operatorname{GL}_{2}$, via a
$\operatorname{GL}_{2}$, via a  $p$-adic Langlands lift attached to the symmetric cube representation. Our proof goes via the comparison of the Galois level with a ‘fortuitous’ congruence ideal. Some of the
$p$-adic Langlands lift attached to the symmetric cube representation. Our proof goes via the comparison of the Galois level with a ‘fortuitous’ congruence ideal. Some of the 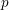 $p$-adic lifts are interpolated by a morphism of rigid analytic spaces from an eigencurve for
$p$-adic lifts are interpolated by a morphism of rigid analytic spaces from an eigencurve for 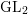 $\operatorname{GL}_{2}$ to an eigenvariety for
$\operatorname{GL}_{2}$ to an eigenvariety for 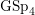 $\operatorname{GSp}_{4}$, while the remainder appear as isolated points on the eigenvariety.
$\operatorname{GSp}_{4}$, while the remainder appear as isolated points on the eigenvariety.
Keywords
- Type
- Research Article
- Information
- Copyright
- © The Author 2019
Footnotes
Current address: Computational Arithmetic Geometry – IWR – Universität Heidelberg, Im Neuenheimer Feld 205, 69120 Heidelberg, Germany
The author was supported by the Programs ArShiFo ANR-10-BLAN-0114 and PerCoLaTor ANR-14-CE25-0002-01.
References
- 1
- Cited by


