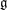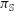Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mărcuţ, Ioan
and
Osorno Torres, Boris
2014.
Deformations of log-symplectic structures.
Journal of the London Mathematical Society,
Vol. 90,
Issue. 1,
p.
197.
Crainic, Marius
Fernandes, Rui Loja
and
Martínez Torres, David
2019.
Poisson manifolds of compact types (PMCT 1).
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2019,
Issue. 756,
p.
101.
Frejlich, Pedro
and
Mărcuț, Ioan
2020.
The Homology Class of a Poisson Transversal.
International Mathematics Research Notices,
Vol. 2020,
Issue. 10,
p.
2952.
Monica Blaga, Adara
Amelia Salazar, Maria
Giuseppe Tortorella, Alfonso
and
Vizman, Cornelia
2020.
Contact Dual Pairs.
International Mathematics Research Notices,
Crooks, Peter
and
Mayrand, Maxence
2022.
Symplectic reduction along a submanifold.
Compositio Mathematica,
Vol. 158,
Issue. 9,
p.
1878.