Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bouchemakh, Isma
and
Engel, Konrad
1997.
The order-interval hypergraph of a finite poset and the König property.
Discrete Mathematics,
Vol. 170,
Issue. 1-3,
p.
51.
Stinson, D.R.
Wei, R.
and
Zhu, L.
2000.
Some New Bounds for Cover-Free Families.
Journal of Combinatorial Theory, Series A,
Vol. 90,
Issue. 1,
p.
224.
D'yachkov, A.G.
Lebedev, V.S.
Vilenkin, P.A.
and
Yekhanin, S.M.
2001.
Cover-free families and superimposed codes: constructions, bounds and applications to cryptography and group testing.
p.
117.
D'yachkov, A.
Vilenkin, P.
Torney, D.
and
Macula, A.
2002.
Families of Finite Sets in which No Intersection of ℓ Sets Is Covered by the Union of s Others.
Journal of Combinatorial Theory, Series A,
Vol. 99,
Issue. 2,
p.
195.
Stinson, D.R.
and
Wei, R.
2004.
Generalized cover-free families.
Discrete Mathematics,
Vol. 279,
Issue. 1-3,
p.
463.
Bouchemakh, Isma
and
Ouatiki, Saliha
2005.
On the domatic and the total domatic numbers of the 2-section graph of the order-interval hypergraph of the finite poset.
Electronic Notes in Discrete Mathematics,
Vol. 22,
Issue. ,
p.
245.
Lebedev, V. S.
2005.
A Note on the Uniqueness of (w, r) Cover-Free Codes.
Problems of Information Transmission,
Vol. 41,
Issue. 3,
p.
199.
Kim, Hyun Kwang
Lebedev, Vladimir
and
Oh, Dong Yeol
2005.
Some new results on superimposed codes.
Journal of Combinatorial Designs,
Vol. 13,
Issue. 4,
p.
276.
Desmedt, Yvo
and
Jakimoski, Goce
2006.
Topics in Cryptology – CT-RSA 2007.
Vol. 4377,
Issue. ,
p.
324.
Chen, H. B.
Du, D. Z.
and
Hwang, F. K.
2007.
An unexpected meeting of four seemingly unrelated problems: graph testing, DNA complex screening, superimposed codes and secure key distribution.
Journal of Combinatorial Optimization,
Vol. 14,
Issue. 2-3,
p.
121.
Hong, S.
Kapralov, S.
Kim, H. K.
and
Oh, D. Y.
2007.
Uniqueness of some optimal superimposed codes.
Problems of Information Transmission,
Vol. 43,
Issue. 2,
p.
113.
Chen, Hong-Bin
Fu, Hung-Lin
and
Hwang, Frank K.
2008.
An upper bound of the number of tests in pooling designs for the error-tolerant complex model.
Optimization Letters,
Vol. 2,
Issue. 3,
p.
425.
Bouchemakh, Isma
and
Ouatiki, Saliha
2009.
On the domatic and the total domatic numbers of the 2-section graph of the order-interval hypergraph of a finite poset.
Discrete Mathematics,
Vol. 309,
Issue. 11,
p.
3674.
Wang, Yongge
and
Desmedt, Yvo
2011.
Information Theoretic Security.
Vol. 6673,
Issue. ,
p.
58.
Hajiabolhassan, Hossein
and
Moazami, Farokhlagha
2012.
Some new bounds for cover-free families through biclique covers.
Discrete Mathematics,
Vol. 312,
Issue. 24,
p.
3626.
2013.
Algebraic Curves in Cryptography.
p.
155.
D’yachkov, A. G.
Vorob’ev, I. V.
Polyansky, N. A.
and
Shchukin, V. Yu.
2014.
Bounds on the rate of disjunctive codes.
Problems of Information Transmission,
Vol. 50,
Issue. 1,
p.
27.
D'yachkov, A. G.
Vorobyev, I.V.
Polyanskii, N.A.
and
Shchukin, V.Yu.
2014.
Bounds on the rate of superimposed codes.
p.
2341.
D'yachkov, A. G.
Vorobyev, I.V.
Polyanskii, N.A.
and
Shchukin, V.Yu.
2015.
Cover-free codes and separating system codes.
p.
2894.
Zhao, Shufang
He, Yichao
Zhang, Xinlu
Xu, Wen
Wu, Weili
and
Gao, Suogang
2016.
Group Testing with Multiple Inhibitor Sets and Error-Tolerant and Its Decoding Algorithms.
Journal of Computational Biology,
Vol. 23,
Issue. 10,
p.
821.
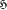 be the hypergraph whose points are the subsets X of [n] := {1,…,n} with l≤ |X| ≤ u, l < u, and whose edges are intervals in the Boolean lattice of the form I = {C ⊆[n] : X⊆C⊆Y} where |X| = l, |Y| = u, X ⊆ Y.We study the matching number
be the hypergraph whose points are the subsets X of [n] := {1,…,n} with l≤ |X| ≤ u, l < u, and whose edges are intervals in the Boolean lattice of the form I = {C ⊆[n] : X⊆C⊆Y} where |X| = l, |Y| = u, X ⊆ Y.We study the matching number 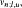 i.e. the the maximum number of pairwise disjoint edges, and the covering number
i.e. the the maximum number of pairwise disjoint edges, and the covering number 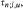 i.e. the minimum number of points which cover all edges. We prove that max
i.e. the minimum number of points which cover all edges. We prove that max  and that for every ε > 0 the inequalities
and that for every ε > 0 the inequalities 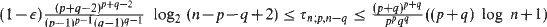 hold, where for the lower bounds we suppose that n is not too small. The corresponding fractional numbers can be determined exactly. Moreover, we show by construction that
hold, where for the lower bounds we suppose that n is not too small. The corresponding fractional numbers can be determined exactly. Moreover, we show by construction that