Article contents
Hamiltonicity in random directed graphs is born resilient
Published online by Cambridge University Press: 24 June 2020
Abstract
Let 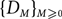 $\{D_M\}_{M\geq 0}$
be the n-vertex random directed graph process, where
$\{D_M\}_{M\geq 0}$
be the n-vertex random directed graph process, where  $D_0$
is the empty directed graph on n vertices, and subsequent directed graphs in the sequence are obtained by the addition of a new directed edge uniformly at random. For each
$D_0$
is the empty directed graph on n vertices, and subsequent directed graphs in the sequence are obtained by the addition of a new directed edge uniformly at random. For each 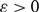 $$\varepsilon > 0$$
, we show that, almost surely, any directed graph
$$\varepsilon > 0$$
, we show that, almost surely, any directed graph 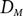 $D_M$
with minimum in- and out-degree at least 1 is not only Hamiltonian (as shown by Frieze), but remains Hamiltonian when edges are removed, as long as at most
$D_M$
with minimum in- and out-degree at least 1 is not only Hamiltonian (as shown by Frieze), but remains Hamiltonian when edges are removed, as long as at most 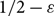 $1/2-\varepsilon$
of both the in- and out-edges incident to each vertex are removed. We say such a directed graph is
$1/2-\varepsilon$
of both the in- and out-edges incident to each vertex are removed. We say such a directed graph is 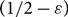 $(1/2-\varepsilon)$
-resiliently Hamiltonian. Furthermore, for each
$(1/2-\varepsilon)$
-resiliently Hamiltonian. Furthermore, for each 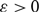 $\varepsilon > 0$
, we show that, almost surely, each directed graph
$\varepsilon > 0$
, we show that, almost surely, each directed graph  $D_M$
in the sequence is not
$D_M$
in the sequence is not 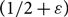 $(1/2+\varepsilon)$
-resiliently Hamiltonian.
$(1/2+\varepsilon)$
-resiliently Hamiltonian.
This improves a result of Ferber, Nenadov, Noever, Peter and Škorić who showed, for each  $\varepsilon > 0$
, that the binomial random directed graph
$\varepsilon > 0$
, that the binomial random directed graph 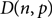 $D(n,p)$
is almost surely
$D(n,p)$
is almost surely  $(1/2-\varepsilon)$
-resiliently Hamiltonian if
$(1/2-\varepsilon)$
-resiliently Hamiltonian if  $p=\omega(\log^8n/n)$
.
$p=\omega(\log^8n/n)$
.
MSC classification
- Type
- Paper
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 5
- Cited by



