Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T19:26:05.761Z Has data issue: false hasContentIssue false
  • English
  • Français

Generalizations of the Ruzsa–Szemerédi and rainbow Turán problems for cliques

Part of: Graph theory

Published online by Cambridge University Press:  19 November 2020

W. T. Gowers  and
Barnabás Janzer
W. T. Gowers
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, Centre for Mathematical Sciences, Wilberforce Road, CambridgeCB3 0WB, UK
Barnabás Janzer*
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, Centre for Mathematical Sciences, Wilberforce Road, CambridgeCB3 0WB, UK
*
*Corresponding author. Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Considering a natural generalization of the Ruzsa–Szemerédi problem, we prove that for any fixed positive integers r, s with r < s, there are graphs on n vertices containing 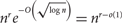$n^{r}e^{-O\left(\sqrt{\log{n}}\right)}=n^{r-o(1)}$ copies of Ks such that any Kr is contained in at most one Ks. We also give bounds for the generalized rainbow Turán problem ex (n, H, rainbow - F) when F is complete. In particular, we answer a question of Gerbner, Mészáros, Methuku and Palmer, showing that there are properly edge-coloured graphs on n vertices with 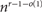$n^{r-1-o(1)}$ copies of Kr such that no Kr is rainbow.

MSC classification

Primary: 05C35: Extremal problems
Secondary: 05C15: Coloring of graphs and hypergraphs
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 30 , Issue 4 , July 2021 , pp. 591 - 608
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2020. Published by Cambridge University Press

References

Alon, N., Lefmann, H. and Rödl, V. (1992) On an anti-Ramsey type result. In Sets, Graphs and Numbers (Budapest, 1991), Vol. 60 of Colloquia Mathematica Societatis János Bolyai, pp. 9–22. North-Holland.Google Scholar
Alon, N., Moitra, A. and Sudakov, B. (2012) Nearly complete graphs decomposable into large induced matchings and their applications. In Proceedings of the Forty-Fourth Annual ACM Symposium on Theory of Computing (STOC ’12), pp. 1079–1090. ACM.CrossRefGoogle Scholar
Alon, N. and Shapira, A. (2006) A characterization of easily testable induced subgraphs. Combin. Probab. Comput. 15 791–805.CrossRefGoogle Scholar
Alon, N. and Shapira, A. (2006) On an extremal hypergraph problem of Brown, Erdös and Sós. Combinatorica 26 627–645.CrossRefGoogle Scholar
Alon, N. and Shikhelman, C. (2016) Many T copies in H-free graphs. J. Combin. Theory Ser. B 121 146–172.CrossRefGoogle Scholar
Behrend, F. A. (1946) On sets of integers which contain no three terms in arithmetical progression. Proc. Nat. Acad. Sci. USA 32 331.CrossRefGoogle Scholar
Das, S., Lee, C. and Sudakov, B. (2013) Rainbow Turán problem for even cycles. Europ. J. Combin. 34 905–915.CrossRefGoogle Scholar
Ergemlidze, B., Győri, E. and Methuku, A. (2019) On the rainbow Turán number of paths. Electron. J. Combin. 26 P1.17.CrossRefGoogle Scholar
Gerbner, D., Mészáros, T., Methuku, A. and Palmer, C. (2019) Generalized rainbow Turán problems. arXiv:1911.06642Google Scholar
Johnston, D., Palmer, C. and Sarkar, A. (2016) Rainbow Turán problems for paths and forests of stars. Electron. J. Combin. 24 P1.34.Google Scholar
Keevash, P., Mubayi, D., Sudakov, B. and Verstraëte, J. (2007) Rainbow Turán problems. Combin. Probab. Comput. 16 109–126.CrossRefGoogle Scholar
Ruzsa, I. Z. and Szemerédi, E. (1979) Triple systems with no six points carrying three triangles. In Combinatorics (Keszthely, 1976), Vol. 18 of Colloquia Mathematica Societatis János Bolyai, pp. 939–945. Akadémiai Kaidó.Google Scholar
Sós, V., Erdős, P. and Brown, W. (1973) On the existence of triangulated spheres in 3-graphs, and related problems. Periodica Math. Hungar. 3 221–228.Google Scholar