Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-28T09:13:31.630Z Has data issue: false hasContentIssue false
  • English
  • Français

Counting Intersecting and Pairs of Cross-Intersecting Families

Part of: Extremal combinatorics

Published online by Cambridge University Press:  16 October 2017

PETER FRANKL  and
ANDREY KUPAVSKII
PETER FRANKL
Affiliation:
Department of Discrete Mathematics, Moscow Institute of Physics and Technology, Moscow, Russia and Ecole Polytechnique Fédérale de Lausanne, Switzerland (e-mail: [email protected])
ANDREY KUPAVSKII
Affiliation:
Department of Discrete Mathematics, Moscow Institute of Physics and Technology, Moscow, Russia and Ecole Polytechnique Fédérale de Lausanne, Switzerland (e-mail: [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

A family of subsets of {1,. . .,n} is called intersecting if any two of its sets intersect. A classical result in extremal combinatorics due to Erdős, Ko and Rado determines the maximum size of an intersecting family of k-subsets of {1,. . .,n}. In this paper we study the following problem: How many intersecting families of k-subsets of {1,. . .,n} are there? Improving a result of Balogh, Das, Delcourt, Liu and Sharifzadeh, we determine this quantity asymptotically for n ≥ 2k+2+2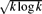$\sqrt{k\log k}$ and k → ∞. Moreover, under the same assumptions we also determine asymptotically the number of non-trivial intersecting families, that is, intersecting families for which the intersection of all sets is empty. We obtain analogous results for pairs of cross-intersecting families.

MSC classification

Primary: 05D05: Extremal set theory
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 27 , Issue 1 , January 2018 , pp. 60 - 68
Copyright
Copyright © Cambridge University Press 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Balogh, J., Das, S. A., Delcourt, M., Liu, H. and Sharifzadeh, M. (2015) Intersecting families of discrete structures are typically trivial. J. Combin. Theory Ser. A 132 224–245.Google Scholar
[2] Bollobás, B. (1965) On generalized graphs. Acta Math. Acad. Sci. Hungar. 16 447–452.Google Scholar
[3] Erdős, P., Ko, C. and Rado, R. (1961) Intersection theorems for systems of finite sets. Quart. J. Math. 12 313–320.Google Scholar
[4] Frankl, P. (1987) Erdős–Ko–Rado theorem with conditions on the maximal degree. J. Combin. Theory Ser. A 46 252–263.CrossRefGoogle Scholar
[5] Frankl, P. and Tokushige, N. (1992) Some best possible inequalities concerning cross-intersecting families. J. Combin. Theory Ser. A 61 87–97.Google Scholar
[6] Hilton, A. J. W. (1976) The Erdős–Ko–Rado theorem with valency conditions. Unpublished manuscript.Google Scholar
[7] Hilton, A. J. W. and Milner, E. C. (1967) Some intersection theorems for systems of finite sets. Quart. J. Math. Oxford 18 369–384.Google Scholar
[8] Jaeger, F. and Payan, C. (1971) Nombre maximal d'arêtes d'un hypergraphe critique de rang h. CR Acad. Sci. Paris 273 221–223.Google Scholar
[9] Katona, G. O. H. (1974) Solution of a problem of A. Ehrenfeucht and J. Mycielski. J. Combin. Theory Ser. A 17 265–266.Google Scholar
[10] Katona, G. O. H. (1987) A theorem of finite sets. In Classic Papers in Combinatorics (Gessel, I. and Rota, G.-C., eds), Birkhäuser, pp. 381–401.Google Scholar
[11] Kruskal, J. B. (1963) The number of simplices in a complex. In Mathematical Optimization Techniques (Bellman, R., ed.), University of California Press, pp. 251–278.Google Scholar
[12] Kupavskii, A. and Zakharov, D. Regular bipartite graphs and intersecting families. Accepted at J. Combin. Theory Ser. A arXiv:1611.03129 Google Scholar
[13] Lovász, L. (1979) Combinatorial Problems and Exercises, North-Holland.Google Scholar