Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T16:58:35.908Z Has data issue: false hasContentIssue false
  • English
  • Français

Counting Independent Sets in Hypergraphs

Published online by Cambridge University Press:  08 April 2014

JEFF COOPER ,
KUNAL DUTTA  and
DHRUV MUBAYI
JEFF COOPER
Affiliation:
Department of Mathematics, Statistics, and Computer Science, University of Illinois at Chicago, IL 60607, USA (e-mail: [email protected], [email protected])
KUNAL DUTTA
Affiliation:
Algorithms and Complexity Department, Max Planck Institute for Informatics, Saarbrücken, Germany (e-mail: [email protected])
DHRUV MUBAYI
Affiliation:
Department of Mathematics, Statistics, and Computer Science, University of Illinois at Chicago, IL 60607, USA (e-mail: [email protected], [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

Let G be a triangle-free graph with n vertices and average degree t. We show that G contains at least

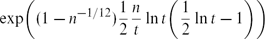${\exp\biggl({1-n^{-1/12})\frac{1}{2}\frac{n}{t}\ln t} \biggl(\frac{1}{2}\ln t-1\biggr)\biggr)}$
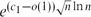independent sets. This improves a recent result of the first and third authors [8]. In particular, it implies that as n → ∞, every triangle-free graph on n vertices has at least ${e^{(c_1-o(1)) \sqrt{n} \ln n}}$ independent sets, where 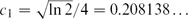$c_1 = \sqrt{\ln 2}/4 = 0.208138 \ldots$. Further, we show that for all n, there exists a triangle-free graph with n vertices which has at most 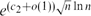${e^{(c_2+o(1))\sqrt{n}\ln n}}$ independent sets, where 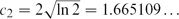$c_2 = 2\sqrt{\ln 2} = 1.665109 \ldots$. This disproves a conjecture from [8].

Let H be a (k+1)-uniform linear hypergraph with n vertices and average degree t. We also show that there exists a constant ck such that the number of independent sets in H is at least

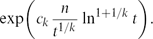${\exp\biggl({c_{k} \frac{n}{t^{1/k}}\ln^{1+1/k}{t}\biggr})}.$
This is tight apart from the constant ck and generalizes a result of Duke, Lefmann and Rödl [9], which guarantees the existence of an independent set of size
$\Omega\biggl(\frac{n}{t^{1/k}} \ln^{1/k}t\biggr).$
 Both of our lower bounds follow from a more general statement, which applies to hereditary properties of hypergraphs.

Keywords

Primary 05D40Secondary 05C6505D05
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 23 , Issue 4 , July 2014 , pp. 539 - 550
Copyright
Copyright © Cambridge University Press 2014 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Ajtai, M., Erdős, P., Komlós, J. and Szemerédi, E. (1981), On Turán's theorem for sparse graphs. Combinatorica 1 313–317.Google Scholar
[2]Ajtai, M., Komlós, J., Pintz, J., Spencer, J. and Szemerédi, E. (1982) Extremal uncrowded hypergraphs. J. Combin. Theory Ser. A 32 321–335.Google Scholar
[3]Ajtai, M., Komlós, J. and Szemerédi, E. (1981) A dense infinite Sidon sequence. Europ. J. Combin. 2 1–11.Google Scholar
[4]Alon, N., Kim, J.-H. and Spencer, J. (1997) Nearly perfect matchings in regular simple hypergraphs. Israel J. Math. 100 171–187.Google Scholar
[5]Asratian, A. S. and Kuzjurin, N. N. (2000) On the number of partial Steiner systems. J. Combin. Des. 8 347–352.Google Scholar
[6]Bohman, T. and Keevash, P. (2013) Dynamic concentration of the triangle-free process. arXiv.1302.5963Google Scholar
[7]Colbourn, C. J., Hoffman, D. G., Phelps, K. T., Rödl, V. and Winkler, P. M. (1991) The number of t-wise balanced designs. Combinatorica 11 207–218.CrossRefGoogle Scholar
[8]Cooper, J. and Mubayi, D. Counting independent sets in triangle-free graphs. Proc. Amer. Math. Soc., to appear.Google Scholar
[9]Duke, R. A., Lefmann, H. and Rödl, V. (1995) On uncrowded hypergraphs. Random Struct. Alg. 6 209–212.Google Scholar
[10]Fiz Pontiveros, G., Griffiths, S. and Morris, R. (2013) The triangle-free process and r(3,k). arXiv.1302.6279Google Scholar
[11]Grable, D. A. and Phelps, K. T. (1996) Random methods in design theory: A survey. J. Combin. Des. 4 255–273.3.0.CO;2-E>CrossRefGoogle Scholar
[12]Kim, J. H. (1995) The Ramsey number R(3,t) has order of magnitude t 2/log t. Random Struct. Alg. 7 173–207.Google Scholar
[13]Kim, J. H. and Vu, V. H. (2000) Concentration of multivariate polynomials and its applications. Combinatorica 20 417–434.CrossRefGoogle Scholar
[14]Molloy, M. and Reed, B. (2002) Graph Colouring and the Probabilistic Method, Vol. 23 of Algorithms and Combinatorics, Springer.Google Scholar
[15]Shearer, J. B. (1983) A note on the independence number of triangle-free graphs. Discrete Math. 46 83–87.CrossRefGoogle Scholar
[16]Shearer, J. B. (1995) On the independence number of sparse graphs. Random Struct. Alg. 7 269–271.Google Scholar