Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kupavskii, Andrey
and
Zakharov, Dmitriy
2018.
Regular bipartite graphs and intersecting families.
Journal of Combinatorial Theory, Series A,
Vol. 155,
Issue. ,
p.
180.
Borg, Peter
2019.
Cross-intersecting non-empty uniform subfamilies of hereditary families.
European Journal of Combinatorics,
Vol. 78,
Issue. ,
p.
256.
Bobu, Andrei Viktorovich
Kupriyanov, Aleksandr Eduardovich
and
Raigorodskii, Andrei Mikhailovich
2020.
Об одном обобщении кнезеровских графов.
Математические заметки,
Vol. 107,
Issue. 3,
p.
351.
Bobu, A. V.
Kupriyanov, A. É.
and
Raigorodskii, A. M.
2020.
A Generalization of Kneser Graphs.
Mathematical Notes,
Vol. 107,
Issue. 3-4,
p.
392.
Gupta, Pranshu
Mogge, Yannick
Piga, Simón
and
Schülke, Bjarne
2021.
Maximum size of r-cross t-intersecting families.
Procedia Computer Science,
Vol. 195,
Issue. ,
p.
453.
Shi, Chao
Frankl, Peter
and
Qian, Jianguo
2022.
On Non-Empty Cross-Intersecting Families.
Combinatorica,
Vol. 42,
Issue. S2,
p.
1513.
Gupta, Pranshu
Mogge, Yannick
Piga, Simón
and
Schülke, Bjarne
2023.
r$r$‐Cross t$t$‐intersecting families via necessary intersection points.
Bulletin of the London Mathematical Society,
Vol. 55,
Issue. 3,
p.
1447.
Xi, Yuanxiao
Kong, Xiangliang
and
Ge, Gennian
2024.
Multi-part cross-intersecting families.
Journal of Algebraic Combinatorics,
Vol. 59,
Issue. 3,
p.
597.
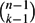 $\binom{n-1}{k-1}$.
$\binom{n-1}{k-1}$. ${\mathcal A}, {\mathcal B}\subset \binom{[n]}{k}$ are s-cross-intersecting if, for any A ∈
${\mathcal A}, {\mathcal B}\subset \binom{[n]}{k}$ are s-cross-intersecting if, for any A ∈ 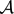 ${\mathcal A}$, B ∈
${\mathcal A}$, B ∈  ${\mathcal B}$, we have |A ∩ B| ≥ s. In this paper we determine the maximum of |
${\mathcal B}$, we have |A ∩ B| ≥ s. In this paper we determine the maximum of |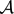 ${\mathcal A}$|+|
${\mathcal A}$|+| ${\mathcal B}$| for all n. This generalizes a result of Hilton and Milner, who determined the maximum of |
${\mathcal B}$| for all n. This generalizes a result of Hilton and Milner, who determined the maximum of |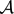 ${\mathcal A}$|+|
${\mathcal A}$|+| ${\mathcal B}$| for non-empty 1-cross-intersecting families.
${\mathcal B}$| for non-empty 1-cross-intersecting families.