No CrossRef data available.
Article contents
Weak Convergence and One-Sample Rank Statistics Under ϕ-mixing*
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let {Xi:i=1, 2,…} be a real strictly stationary process (defined on a probability space (Ω, A, P)) which has absolutely continuous finite dimensional distributions (with respect to Lebesgue measure) and satisfies the ϕ-mixing condition: Let 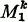 and
and  denote the sub-cr-fields generated, respectively, by {Xi:i≤k} and {Xi:i≥k+n}; then, for each k≥1 and n≥l,
denote the sub-cr-fields generated, respectively, by {Xi:i≤k} and {Xi:i≥k+n}; then, for each k≥1 and n≥l,  and
and 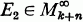 together imply.
together imply.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1975
Footnotes
*
Supported partially by the National Research Council of Canada Grant #A-3061.
References
1.
Billingsley, Patrick, Weak convergence of probability measures, John Wiley & Sons, Inc., New York, 1968.Google Scholar
2.
Chernoff, H. and Savage, I. R.
Asymptotic normality and efficiency of certain nonparametric test statistics, Ann. Math. Statist.,
29 (1958), 972–994.Google Scholar
3.
Fears, T. R. and Mehra, K. L., Weak convergence of a two-sample empirical process and a Chernoff-Savage Theorem for ϕ-mixing sequences, Ann. Statist.,
2 (1974), 586–596.Google Scholar
4.
Pyke, R. and Shorack, G., Weak convergence of two-sample empirical process and a new approach to Chernoff-Savage theorems, Ann. Math. Statist.,
39 (1968), 755–771.Google Scholar
5.
Pyke, R. and Shorack, G.
Weak convergence and a Chernoff-Savage theorem for random sample sizes, Ann. Math. Statist.,
39 (1968), 1675–1685.Google Scholar
6.
Pyke, R. and Shorack, G., A note on Chernoff-Savage theorems, Ann. Math. Statist.,
40 (1969), 1116–1119.Google Scholar


