Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Tingley, Daryl
1984.
Noncontractive uniformly Lipshitzian semigroups in Hilbert space.
Proceedings of the American Mathematical Society,
Vol. 92,
Issue. 3,
p.
355.
Ishihara, Hajime
and
Takahashi, Wataru
1987.
Modulus of convexity, characteristic of convexity and fixed point theorems.
Kodai Mathematical Journal,
Vol. 10,
Issue. 2,
Ishihara, Hajime
and
Takahashi, Wataru
1987.
Fixed point theorems for uniformly lipschitzian semigroups in Hilbert spaces.
Journal of Mathematical Analysis and Applications,
Vol. 127,
Issue. 1,
p.
206.
Mizoguchi, Noriko
and
Takahashi, Wataru
1990.
On the existence of fixed points and ergodic retractions for Lipschitzian semigroups in Hilbert spaces.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 14,
Issue. 1,
p.
69.
Xu, Hong-Kun
1990.
Fixed point theorems for uniformly Lipschitzian semigroups in uniformly convex spaces.
Journal of Mathematical Analysis and Applications,
Vol. 152,
Issue. 2,
p.
391.
Tan, Kok-Keong
and
Xu, Hong-Kun
1993.
Fixed point theorems for Lipschitzian semigroups in Banach spaces.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 20,
Issue. 4,
p.
395.
Zeng, Lu-Chuan
1995.
On the existence of fixed points and nonlinear ergodic retractions for Lipschitzian semigroups without convexity.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 24,
Issue. 9,
p.
1347.
Górnicki, Jaroslaw
1997.
Lipschitzian semigroups in Hilbert space.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 30,
Issue. 4,
p.
2309.
Lau, Anthony To-Ming
and
Takahashi, Wataru
2001.
Handbook of Metric Fixed Point Theory.
p.
517.
Song, Xueli
and
Peng, Jigen
2010.
Lipschitzian semigroups and abstract functional differential equations.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 72,
Issue. 5,
p.
2346.
Mei, Zhan-Dong
Peng, Ji-Gen
and
Song, Xue-Li
2011.
A class of semilinear systems with state and input delays.
Journal of Dynamical and Control Systems,
Vol. 17,
Issue. 1,
p.
123.
Marom, Saiful
and
Istiqlal, Muhammad
2023.
Fixed Point Theorems for Semigroups of Lipschitzian Mappings.
Journal of Multidisciplinary Applied Natural Science,
Vol. 4,
Issue. 1,
p.
90.


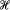 . It is shown that if
. It is shown that if 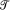 is a left reversible, uniformly
is a left reversible, uniformly 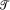 has a common fixed point in
has a common fixed point in 