Ternary Quadratic Forms and Eta Quotients

Abstract

Let $\eta \left( z \right)\,\left( z\,\in \,\mathbb{C},\,\operatorname{Im}\left( z \right)\,>\,0 \right)$ denote the Dedekind eta function. We use a recent product-to-sum formula in conjunction with conditions for the non-representability of integers by certain ternary quadratic forms to give explicitly ten eta quotients

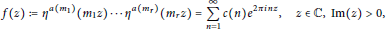$$f\left( z \right)\,:=\,{{\eta }^{a\left( {{m}_{1}} \right)}}\,\left( {{m}_{1}}z \right)\,.\,.\,.\,{{\eta }^{a\left( {{m}_{r}} \right)}}\,\left( {{m}_{r}}z \right)\,=\,\sum\limits_{n=1}^{\infty }{c\left( n \right){{e}^{2\pi inz}},\,\,\,z\,\in \,\mathbb{C},\,\operatorname{Im}\left( z \right)\,>\,0,}$$

such that the Fourier coefficients 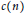$c\left( n \right)$ vanish for all positive integers $n$ in each of infinitely many non-overlapping arithmetic progressions. For example, we show that if 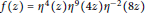$f\left( z \right)\,=\,{{\eta }^{4}}\left( z \right){{\eta }^{9}}\left( 4z \right){{\eta }^{-2}}\left( 8z \right)$ we have 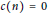$c\left( n \right)\,=\,0$ for all $n$ in each of the arithmetic progressions 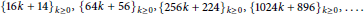${{\{16k\,+\,14\}}_{k\ge 0}},\,{{\{64k\,+\,56\}}_{k\ge 0}},\,{{\{256k,\,224\}}_{k\ge 0}},\,{{\{1024k\,+\,869\}}_{k\ge 0}},\,.\,.\,.$