Hostname: page-component-745bb68f8f-grxwn Total loading time: 0 Render date: 2025-02-03T16:22:06.458Z Has data issue: false hasContentIssue false
  • English
  • Français

Spectra of Boolean Graphs Over Finite Fields of Characteristic Two

Part of: Finite commutative rings Graph theory

Published online by Cambridge University Press:  04 November 2019

D. Scott Dillery  and
John D. LaGrange
D. Scott Dillery
Affiliation:
School of Mathematics and Sciences, Lindsey Wilson College, Columbia, KY 42728-1223, USA Email: [email protected]@lindsey.edu
John D. LaGrange
Affiliation:
School of Mathematics and Sciences, Lindsey Wilson College, Columbia, KY 42728-1223, USA Email: [email protected]@lindsey.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

With entries of the adjacency matrix of a simple graph being regarded as elements of 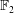$\mathbb{F}_{2}$, it is proved that a finite commutative ring 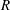$R$ with 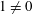$1\neq 0$ is a Boolean ring if and only if either 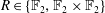$R\in \{\mathbb{F}_{2},\mathbb{F}_{2}\times \mathbb{F}_{2}\}$ or the eigenvalues (in the algebraic closure of 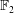$\mathbb{F}_{2}$) corresponding to the zero-divisor graph of $R$ are precisely the elements of $\mathbb{F}_{4}\setminus \{0\}$ . This is achieved by observing a way in which algebraic behavior in a Boolean ring is encoded within Pascal’s triangle so that computations can be carried out by appealing to classical results from number theory.

Keywords

zero-divisor graphBoolean ringeigenvaluePascal matrix

MSC classification

Primary: 05C25: Graphs and abstract algebra (groups, rings, fields, etc.)
Secondary: 05C50: Graphs and linear algebra (matrices, eigenvalues, etc.) 13M99: None of the above, but in this section
Type
Article
Information
Canadian Mathematical Bulletin , Volume 63 , Issue 1 , March 2020 , pp. 58 - 65
Copyright
© Canadian Mathematical Society 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, D. F. and Livingston, P. S., The zero-divisor graph of a commutative ring. J. Algebra 217(1999), 434–447. https://doi.org/10.1006/jabr.1998.7840Google Scholar
Atiyah, M. F. and MacDonald, I. G., Introduction to commutative algebra. Addison-Wesley, Reading, MA, 1969.Google Scholar
Barik, S., Neumann, M., and Pati, S., On nonsingular trees and a reciprocal eigenvalue property. Linear Multilinear Algebra 54(2006), 453–465. https://doi.org/10.1080/03081080600792897Google Scholar
Carlitz, L., The characteristic polynomial of a certain matrix of binomial coefficients. Fibonacci Quart. 3(1965), 81–89.Google Scholar
Cvetković, D. M., Doob, M., and Sachs, H., Spectra of graphs. Theory and application. Pure and Applied Mathematics, 87, Academic Press, New York, 1979.Google Scholar
Fine, N. J., Binomial coefficients modulo a prime. Amer. Math. Monthly 54(1947), 589–592. https://doi.org/10.2307/2304500Google Scholar
Godsil, C. and Royle, G., Algebraic graph theory. Graduate Texts in Mathematics, 207, Springer-Verlag, New York, 2001.Google Scholar
LaGrange, J. D., A combinatorial development of Fibonacci numbers in graph spectra. Linear Algebra Appl. 438(2013), 4335–4347.Google Scholar
LaGrange, J. D., Boolean rings and reciprocal eigenvalue properties. Linear Algebra Appl. 436(2012), 1863–1871. https://doi.org/10.1016/j.laa.2011.05.042Google Scholar
LaGrange, J. D., Eigenvalues of Boolean graphs and Pascal-type matrices. Int. Electron. J. Algebra 13(2013), 109–119.Google Scholar
Lu, D. and Wu, T., The zero-divisor graphs which are uniquely determined by neighborhoods. Comm. Algebra 35(2007), 3855–3864. https://doi.org/10.1080/00927870701509156Google Scholar
Panda, S. K. and Pati, S., On some graphs which satisfy reciprocal eigenvalue properties. Linear Algebra Appl. 530(2017), 445–460. https://doi.org/10.1016/j.laa.2017.04.017Google Scholar