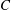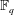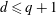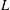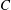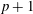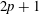Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Asgarli, Shamil
Duan, Lian
and
Lai, Kuan-Wen
2021.
Transverse lines to surfaces over finite fields.
manuscripta mathematica,
Vol. 165,
Issue. 1-2,
p.
135.
Asgarli, Shamil
and
Freidin, Brian
2021.
On the proportion of transverse-free plane curves.
Finite Fields and Their Applications,
Vol. 72,
Issue. ,
p.
101833.
Asgarli, Shamil
and
Ghioca, Dragos
2022.
A Bertini type theorem for pencils over finite fields.
Finite Fields and Their Applications,
Vol. 77,
Issue. ,
p.
101936.
Asgarli, Shamil
Duan, Lian
and
Lai, Kuan-Wen
2024.
Transverse linear subspaces to hypersurfaces over finite fields.
Finite Fields and Their Applications,
Vol. 95,
Issue. ,
p.
102396.