No CrossRef data available.
Article contents
Sequences with Translates Containing Many Primes
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Garrison [3], Forman [2], and Abel and Siebert [1] showed that for all positive integers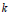 
$k$ and 
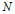$N$, there exists a positive integer 
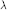$\lambda $ such that 
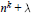${{n}^{k}}\,+\,\lambda $ is prime for at least 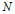$N$ positive integers 
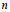$n$. In other words, there exists 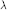$\lambda $ such that 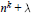${{n}^{k}}\,+\,\lambda $, represents at least 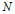$N$ primes.
We give a quantitative version of this result.We show that there exists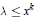 
$\lambda \le {{x}^{k}}$ such that 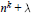${{n}^{k}}\,+\,\lambda $, 1 ≤ n ≤ x, represents at least 
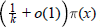$\left( \frac{1}{k}\,+\,o\left( 1 \right) \right)\,\pi \left( x \right)$ primes, as 
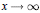$x\to \infty $. We also give some related results.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1998
References
1.
Abel, V. and Siebert, H., Sequences with large numbers of prime values. Amer.Math.Monthly 100 (1993), 167–169.Google Scholar
3.
Garrison, B., Polynomials with large numbers of prime values. Amer.Math.Monthly 97 (1990), 316–317.Google Scholar
5.
Sierpinski, W., Les binomes x2 + n et les nombres premiers. Bull. Soc. Roy. Sci. Liége 33 (1964), 259–260.Google Scholar
You have
Access