Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T20:23:56.342Z Has data issue: false hasContentIssue false
  • English
  • Français

Self-Converse Tournaments

Published online by Cambridge University Press:  20 November 2018

W. J. R. Eplett
W. J. R. Eplett*
Affiliation:
Department of MathematicsThe University of Reading Whiteknights, Reading RG6 2AX, England
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

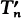Let Tn denote a tournament with vertices labelled 1, …, n. Any undefined terms can be found in [5]. The converse of Tn is the tournament obtained by reversing the orientation of all the arcs in 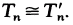Tn. A tournament is called self-converse (s.c.) if . The transitive tournaments are examples of s.c. tournaments. In this paper we provide a structural characterization of s.c. tournaments and we also characterize the score vectors of s.c. tournaments.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 22 , Issue 1 , 01 March 1979 , pp. 23 - 27
Copyright
Copyright © Canadian Mathematical Society 1979

References

1. Brauer, A., Gentry, I. C. and Shaw, K., A new proof of a theorem by H. G. Landau on tournament matrices, J. Combinatorial Theory 5 (1968), 289-292.Google Scholar
2. Hall, M., The Theory of Groups, Macmillan, New York, 1970.Google Scholar
3. Harary, F. and Palmer, E. M., Graphical Enumeration, Academic Press, New York, 1973.Google Scholar
4. Landau, H. G., On dominance relations and the structure of animal societies III: the conditions for a score structure, Bull. Math. Biophys, 15 (1953), 143-148.Google Scholar
5. Moon, J. W., Topics on Tournaments, Holt, Rinehart and Winston, New York, 1968.Google Scholar