Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T20:35:54.306Z Has data issue: false hasContentIssue false
  • English
  • Français

The Ring Scheme Fg

Published online by Cambridge University Press:  20 November 2018

Ian G. Connell
Ian G. Connell*
Affiliation:
McGill University, Montreal, Quebec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let A be a ring and s2(A) the set of idempotents in A. It is a familiar fact that s2(A) becomes a ring if we define 0, 1 and multiplication as in A, and a new negative and new addition by

1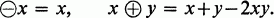

R. A. Melter [3] made the surprising observation that s3(A), where in general sq(A)={x∈A:xq=x}, is a ring if 2 is a unit in A and we define 0,1 minus and multiplication as in A, and a new addition by

2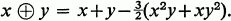

The nonobvious facts are that s3(A) is closed under ⊕ and that ⊕ is associative when applied to the elements of s3(A). The ⊕ in (1) is actually a formal group over A, and so is associative when applied to any elements of A. However in (2) (and similarly in other cases we shall define) the ⊕ is not a formal group, and the associative law depends on the fact that the elements involved are in s3(A).

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 15 , Issue 1 , March 1972 , pp. 79 - 85
Copyright
Copyright © Canadian Mathematical Society 1972

References

1. Demazure, M. and Grothendieck, A., Schémas en Groupes, Fasc. 1, Séminaire de Géométrie Algébrique , Inst. Hautes études Sci. Publ. Math., Paris, 1963.Google Scholar
2. Hasse, H., Vorlesungen tiber Zahlentheorie, Springer-Verlag, Berlin, 1950.Google Scholar
3. Melter, R. A., Problem for solution No. 104 , Canad. Math. Bull. (5) 8 (1965), p. 669.Google Scholar
4. Weil, A., Jacobi Sums as "Grössencharaktere" , Trans. Amer. Math. Soc. 73 (1952), 487-495.Google Scholar