Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-28T05:00:33.644Z Has data issue: false hasContentIssue false
  • English
  • Français

Rigidity of Hamiltonian Actions

Published online by Cambridge University Press:  20 November 2018

Frédéric Rochon
Frédéric Rochon*
Affiliation:
Département de mathématiques, Université du Québec à Montréal, Québec, Canada
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper studies the following question: Given an 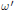${\omega }'$-symplectic action of a Lie group on a manifold $M$ which coincides, as a smooth action, with a Hamiltonian 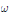$\omega$-action, when is this action a Hamiltonian 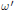${\omega }'$-action? Using a result of Morse-Bott theory presented in Section 2, we show in Section 3 of this paper that such an action is in fact a Hamiltonian 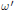${\omega }'$-action, provided that $M$ is compact and that the Lie group is compact and connected. This result was first proved by Lalonde-McDuff-Polterovich in 1999 as a consequence of a more general theory that made use of hard geometric analysis. In this paper, we prove it using classical methods only.

Keywords

53D0537J25
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 46 , Issue 2 , 01 June 2003 , pp. 277 - 290
Copyright
Copyright © Canadian Mathematical Society 2003

References

[1] Bröcker, T. and Dieck, T. T., Representations of Compact Lie Groups. Graduate Texts in Math. 98, Springer-Verlag, New York, 1985.Google Scholar
[2] Hartman, P., Ordinary differential equations. Wiley, New York, 1964.Google Scholar
[3] Lalonde, F. and McDuff, D., Cohomological properties of ruled symplectic structures. In: Mirror symmetry and string geometry, CRM Lecture Notes and Proceedings (eds. E. D'Hoker, D. Phong and S. T. Yau), Proceedings of the workshop onMirror symmetry and string geometry (March 2000, CRM, Montreal), American Mathematical Society, 2001, to appear.Google Scholar
[4] McDuff, D. and Salamon, D., Introduction to Symplectic Topology. Oxford Science Publications, 1995.Google Scholar
[5] Milnor, J., Morse Theory. Ann. of Math. Stud. 51, Princeton University Press, 1963.Google Scholar
[6] Quan, Pham Mau, Introduction à la géométrie des variétés différentiables. Dunod, Paris, 1969.Google Scholar