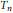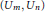Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yamagishi, Masakazu
2015.
Resultants of Chebyshev Polynomials: the First, Second, Third, and Fourth Kinds.
Canadian Mathematical Bulletin,
Vol. 58,
Issue. 2,
p.
423.
Loper, K. Alan
and
Werner, Nicholas J.
2016.
Resultants of minimal polynomials of maximal real cyclotomic extensions.
Journal of Number Theory,
Vol. 158,
Issue. ,
p.
298.
Yamagata, Koji
and
Yamagishi, Masakazu
2018.
Resultants and discriminants of the multiplication polynomials of Jacobi elliptic functions.
Journal of Number Theory,
Vol. 186,
Issue. ,
p.
147.
Wang, Wei
Yan, Zhidan
and
Mao, Lihuan
2024.
Proof of a conjecture on the determinant of the walk matrix of rooted product with a path.
Linear and Multilinear Algebra,
Vol. 72,
Issue. 5,
p.
828.