Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T21:59:15.816Z Has data issue: false hasContentIssue false
  • English
  • Français

A Residue Formula for 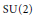$\text{SU(2)}$-Valued Moment Maps

Published online by Cambridge University Press:  20 November 2018

Olga Plamenevskaya
Olga Plamenevskaya*
Affiliation:
Harvard University Department of Mathematics MIT Cambridge, MA 02139 U.S.A., e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Jeffrey and Kirwan gave expressions for intersection pairings on the reduced space 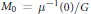${{M}_{0\,}}=\,{{\mu }^{-1}}(0)/G$ of a Hamiltonian $G$-space $M$ in terms of multiple residues. In this paper we prove a residue formula for symplectic volumes of reduced spaces of a quasi-Hamiltonian 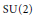$\text{SU(2)}$-space. The definition of quasi-Hamiltonian $G$-spaces was introduced by Alekseev, Malkin and Meinrenken.

Keywords

58F05
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 47 , Issue 4 , 01 December 2004 , pp. 607 - 614
Copyright
Copyright © Canadian Mathematical Society 2004

References

[1] Alekseev, A., Malkin, A. and Meinrenken, E., Lie group valued moment maps. J. Differential Geom. 48 (1998), 445–495.Google Scholar
[2] Alekseev, A., Meinrenken, E. and Woodward, C., Group valued equivariant localization. Invent.Math. 140 (2000), 327–350.Google Scholar
[3] Alekseev, A., Meinrenken, E. and Woodward, C., Duistermaat-Heckman measures and moduli spaces of flat bundles over surfaces. Geom. Funct. Anal. 12 (2002), 1–31.Google Scholar
[4] Berline, N. and Vergne, M., Classes charactéristiques équivariantes. Formules de localisation en cohomologie équivariante, C. R. Acad. Sci. Paris 295(1982) 539–541.Google Scholar
[5] Jeffrey, L., and Kirwan, F., Localization for Nonabelian Group Actions. Topology 34 (1995), 291–327.Google Scholar
[6] Jeffrey, L., and Kirwan, F., Intersection pairings in moduli spaces of holomorphic bundles on a Riemann surface. Electron. Res. Announc. Amer.Math. Soc. 1 (1995), 57–71.Google Scholar
[7] Szenes, A., Iterated Residues and Multiple Bernoulli Polynomials, Internat.Math. Res. Notices 1998, 937–956.Google Scholar
[8] Witten, E., Two-dimensional gauge theories revisited. J. Geom. Phys. 9 (1992), 303–368.Google Scholar