Representation Equivalent Bieberbach Groups and Strongly Isospectral Flat Manifolds

Abstract

Let 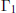${{\Gamma }_{1}}$ and 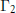${{\Gamma }_{2}}$ be Bieberbach groups contained in the full isometry group $G$ of ${{\mathbb{R}}^{n}}$. We prove that if the compact flat manifolds 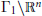${{\Gamma }_{1}}\backslash {{\mathbb{R}}^{n}}$ and ${{\Gamma }_{2}}\backslash {{\mathbb{R}}^{n}}$ are strongly isospectral, then the Bieberbach groups 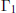${{\Gamma }_{1}}$ and 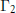${{\Gamma }_{2}}$ are representation equivalent; that is, the right regular representations 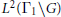${{L}^{2}}\left( {{\Gamma }_{1}}\backslash G \right)$ and 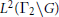${{L}^{2}}\left( {{\Gamma }_{2}}\backslash G \right)$ are unitarily equivalent.