Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-26T18:49:27.042Z Has data issue: false hasContentIssue false
  • English
  • Français

Ramanujan and the Modular j-Invariant

Published online by Cambridge University Press:  20 November 2018

Bruce C. Berndt  and
Heng Huat Chan
Bruce C. Berndt
Affiliation:
Department of Mathematics, University of Illinois, 1409 West Green Street, Urbana, IL 61801 USA
Heng Huat Chan
Affiliation:
Department of Mathematics, National University of Singapore, Kent Ridge, Singapore 119260, Republic of Singapore
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

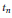A new infinite product ${{t}_{n}}$ was introduced by S. Ramanujan on the last page of his third notebook. In this paper, we prove Ramanujan’s assertions about 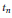${{t}_{n}}$ by establishing new connections between the modular 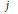$j$-invariant and Ramanujan’s cubic theory of elliptic functions to alternative bases. We also show that for certain integers 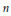$n$, 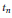${{t}_{n}}$ generates the Hilbert class field of $\mathbb{Q}\left( \sqrt{-n} \right)$. This shows that 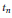${{t}_{n}}$ is a new class invariant according to H. Weber’s definition of class invariants.

Keywords

33C0533E0511R2011R29modular functionsthe Borweins’ cubic theta-functionsHilbert class fields
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 42 , Issue 4 , 01 December 1999 , pp. 427 - 440
Copyright
Copyright © Canadian Mathematical Society 1999

References

[1] Berndt, B. C., Ramanujan's Notebooks, Part III. Springer-Verlag, New York, 1991.Google Scholar
[2] Berndt, B. C., Ramanujan's Notebooks, Part V. Springer-Verlag, New York, 1998.Google Scholar
[3] Berndt, B. C., Bhargava, S. and Garvan, F. G., Ramanujan's theories of elliptic functions to alternative bases. Trans. Amer.Math. Soc. 347 (1995), 4163–4244.Google Scholar
[4] Berndt, B. C., Chan, H. H. and Zhang, L.-C., Ramanujan's class invariants, Kronecker's limit formula, and modular equations. Trans. Amer.Math. Soc. 349 (1997), 2125–2173.Google Scholar
[5] Berndt, B. C. and Rankin, R. A., Ramanujan: Letters and Commentary. American Mathematical Society, Providence, RI; London Mathematical Society, London, 1995.Google Scholar
[6] Berwick, W. E., Modular invariants expressible in terms of quadratic and cubic irrationalities. Proc. London Math. Soc. 28 (1927), 53–69.Google Scholar
[7] Birch, B. J., Weber's class invariants. Mathematika 16 (1969), 283–294.Google Scholar
[8] Borwein, J. M. and Borwein, P. B., Pi and the AGM. John Wiley, New York, 1987.Google Scholar
[9] Borwein, J. M. and Borwein, P. B., A cubic counterpart of Jacobi's identity and the AGM. Trans. Amer.Math. Soc. 323 (1991), 691–701.Google Scholar
[10] Borwein, J. M., Borwein, P. B. and Garvan, F. G., Some cubic identities of Ramanujan. Trans. Amer.Math. Soc. 343 (1994), 35–47.Google Scholar
[11] Chan, H. H., On Ramanujan's cubic transformation formula for 2f1(1/3, 2/3; 1;z). Math. Proc. Camb. Phil. Soc. 124 (1998), 193–204.Google Scholar
[12] Chan, H. H. and Lang, M.-L., On Ramanujan's modular equations and Atkin-Lehner involutions. Israel J. Math. 103 (1998), 1–16.Google Scholar
[13] Chan, H. H. and Liaw, W.-C., On Russell type modular equations. Canad. J. Math., to appear.Google Scholar
[14] Chandrasekharan, K., Elliptic Functions. Springer-Verlag, Berlin, 1985.Google Scholar
[15] Cox, D. A., Primes of the form x2 + ny2 . Wiley, New York, 1989.Google Scholar
[16] Deuring, M., Die Klassenk¨orper der komplexen Multiplikation. Enz. Math. Wiss. Band I2, Heft 10 Teil II, Stuttgart, 1958.Google Scholar
[17] Greenhill, A. G., Complex multiplication moduli of elliptic functions. Proc. London Math. Soc. 19(1887–88), 301–364.Google Scholar
[18] Greenhill, A. G., Table of complex multiplication moduli. Proc. London Math. Soc. 21(1889–90), 403–422.Google Scholar
[19] Greenhill, A. G., The Applications of Elliptic Functions. Dover, New York, 1959.Google Scholar
[20] Newman, M., Construction and application of a class of modular functions II. Proc. London Math. Soc. 9 (1959), 373–387.Google Scholar
[21] Ramanujan, S., Notebooks. 2 vols., Tata Institute of Fundamental Research, Bombay, 1957.Google Scholar
[22] Russell, R., On kλ − k′λ′ modular equations. Proc. London Math. Soc. 19 (1887), 90–111.Google Scholar
[23] Russell, R., On modular equations. Proc. LondonMath. Soc. 21 (1890), 351–395.Google Scholar
[24] S¨ohngen, H., Zur komplexen Multiplikation.Math. Ann. 111 (1935), 302–328.Google Scholar
[25] Stark, H. M., Class numbers of complex quadratic fields. In: Modular functions of one variable I (ed. W. Kuijk), Lecture Notes in Math. 320(1973), Springer-Verlag, 154–174.Google Scholar
[26] Weber, H., Lehrbuch der Algebra, dritter Band. Chelsea, New York, 1961.Google Scholar