Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-29T04:22:52.288Z Has data issue: false hasContentIssue false
  • English
  • Français

A Property of Lie Group Orbits

Published online by Cambridge University Press:  20 November 2018

Mladen Božičević
Mladen Božičević*
Affiliation:
University of Zagreb Department of Geotechnics Hallerova aleja 7 42000 Varaždin Croatia email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 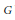$G$ be a real Lie group and 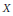$X$ a real analytic manifold. Suppose that 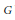$G$ acts analytically on 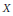$X$ with finitely many orbits. Then the orbits are subanalytic in 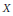$X$. As a consequence we show that the micro-support of a 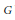$G$-equivariant sheaf on 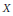$X$ is contained in the conormal variety of the 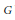$G$-action.

Keywords

32B2022E15
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 43 , Issue 1 , 01 March 2000 , pp. 47 - 50
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Borho, W. and Brylinski, J.-L., Differential operators on homogeneous spaces III. Invent.Math. 80 (1985), 1–61.Google Scholar
[2] Bierstone, E. and Milman, P. D., Semi-analytic and subanalytic sets. Inst. Hautes Études Sci. Publ. Math. 67 (1988), 5–42.Google Scholar
[3] Kashiwara, M. and Schapira, P., Sheaves on Manifolds. Springer-Verlag, 1990.Google Scholar
[4] Warner, G., Harmonic Analysis on Semi-Simple Lie Groups I. Springer-Verlag, 1972.Google Scholar