Hostname: page-component-6bf8c574d5-fppsw Total loading time: 0 Render date: 2025-02-14T12:19:04.857Z Has data issue: false hasContentIssue false
  • English
  • Français

Product decompositions of moment-angle manifolds and B-rigidity

Part of: Arithmetic rings and other special rings Applied homological algebra and category theory

Published online by Cambridge University Press:  15 May 2023

Steven Amelotte Open the ORCID record for Steven Amelotte [Opens in a new window]  and
Benjamin Briggs Open the ORCID record for Benjamin Briggs [Opens in a new window]
Steven Amelotte*
Affiliation:
Institute for Computational and Experimental Research in Mathematics, Brown University, Providence, RI 02903, USA
Benjamin Briggs
Affiliation:
Mathematical Sciences Research Institute, 17 Gauss Way, Berkeley, CA 94720, USA e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A simple polytope P is called B-rigid if its combinatorial type is determined by the cohomology ring of the moment-angle manifold 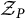$\mathcal {Z}_P$ over P. We show that any tensor product decomposition of this cohomology ring is geometrically realized by a product decomposition of the moment-angle manifold up to equivariant diffeomorphism. As an application, we find that B-rigid polytopes are closed under products, generalizing some recent results in the toric topology literature. Algebraically, our proof establishes that the Koszul homology of a Gorenstein Stanley–Reisner ring admits a nontrivial tensor product decomposition if and only if the underlying simplicial complex decomposes as a join of full subcomplexes.

Keywords

Moment-angle complexcohomological rigidityStanley–Reisner ringquasitoric manifold

MSC classification

Primary: 13F55: Stanley-Reisner face rings; simplicial complexes 55U10: Simplicial sets and complexes
Type
Article
Information
Canadian Mathematical Bulletin , Volume 66 , Issue 4 , December 2023 , pp. 1313 - 1325
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of The Canadian Mathematical Society

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

During this work, the first author was hosted by the Institute for Computational and Experimental Research in Mathematics in Providence, RI, supported by the National Science Foundation under Grant No. 1929284. The second author was hosted by the Mathematical Sciences Research Institute in Berkeley, CA, supported by the National Science Foundation under Grant No. 1928930.

References

Avramov, L. L. and Golod, E. S., Homology algebra of the Koszul complex of a local Gorenstein ring . Math. Notes 9(1971), 30–32.CrossRefGoogle Scholar
Bosio, F., Two transformations of simple polytopes preserving moment-angle manifolds. Preprint, 2017. arXiv:1708.00399Google Scholar
Bruns, W. and Herzog, H. J., Cohen–Macaulay rings, Cambridge Studies in Advanced Mathematics, 39, Cambridge University Press, Cambridge, 1998.CrossRefGoogle Scholar
Buchstaber, V. M., Lectures on toric topology . Trends Math. 10(2008), no. 1, 1–64.Google Scholar
Buchstaber, V. M., Erokhovets, N. Y., Masuda, M., Panov, T. E., and Park, S., Cohomological rigidity of manifolds defined by right-angled 3-dimensional polytopes . Russian Math. Surveys 72(2017), no. 2, 199–256.CrossRefGoogle Scholar
Buchstaber, V. M. and Panov, T. E., Toric topology, Mathematical Surveys and Monographs, 204, American Mathematical Society, Providence, RI, 2015.CrossRefGoogle Scholar
Cai, L., On products in a real moment-angle manifold . J. Math. Soc. Japan 69(2017), no. 2, 503–528.CrossRefGoogle Scholar
Choi, S. and Kim, J. S., Combinatorial rigidity of 3-dimensional simplicial polytopes . Int. Math. Res. Not. 8(2011), 1935–1951.Google Scholar
Choi, S., Panov, T. E., and Suh, D. Y., Toric cohomological rigidity of simple convex polytopes . J. London Math. Soc. 82(2010), no. 2, 343–360.CrossRefGoogle Scholar
Choi, S. and Park, K., Example of C-rigid polytopes which are not B-rigid . Math. Slovaca 69(2019), no. 2, 437–448.CrossRefGoogle Scholar
Choi, S. and Park, S., Projective bundles over toric surfaces . Internat. J. Math. 27(2016), no. 4, 1650032.CrossRefGoogle Scholar
Davis, M. W. and Januszkiewicz, T., Convex polytopes, coxeter orbifolds and torus actions . Duke Math. J. 62(1991), no. 2, 417–451.CrossRefGoogle Scholar
Eisenbud, D., Commutative algebra with a view toward algebraic geometry, Graduate Texts in Mathematics, 150, Springer, New York, 1995.Google Scholar
Erokhovets, N. Y., B-rigidity of ideal almost Pogorelov polytopes . In: Rokhlin-Memorial, V. A., Buchstaber, A. V. M., Malyutin, A. V., and Vershik, A. M. (eds.), Topology, geometry, and dynamics, Contemporary Mathematics, 772, American Mathematical Society, Providence, RI, 2021, pp. 107–122.CrossRefGoogle Scholar
Fan, F., Ma, J., and Wang, X., B-rigidity of flag 2-spheres without 4-belt. Preprint, 2015. arXiv:1511.03624 Google Scholar
Fan, F., Ma, J., and Wang, X., Some rigidity problems in toric topology: I. Preprint, 2020. arXiv:2004.03362 Google Scholar
Hochster, M., Cohen–Macaulay rings, combinatorics, and simplicial complexes . In: McDonald, B. R. and Morris, R. (eds.), Ring theory II (Proc. Second Oklahoma Conference). Dekker, New York, 1977, pp. 171–223.Google Scholar
Mundet i Riera, I., Lifts of smooth group actions to line bundles . Bull. Lond. Math. Soc. 33(2001), 351–361.CrossRefGoogle Scholar
Stanley, R., Combinatorics and commutative algebra , 2nd ed., Progress in Mathematics, 41, Birkhäuser, Boston, MA, 1996.Google Scholar