No CrossRef data available.
Article contents
Poincaré Lemma on Quaternion-like Heisenberg Groups
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
For smooth functions 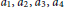 ${{a}_{1}}\,,\,{{a}_{2}}\,,\,{{a}_{3}}\,,\,{{a}_{4}}\,$ on a quaternion Heisenberg group, we characterize the existence of solutions of the partial differential operator system
${{a}_{1}}\,,\,{{a}_{2}}\,,\,{{a}_{3}}\,,\,{{a}_{4}}\,$ on a quaternion Heisenberg group, we characterize the existence of solutions of the partial differential operator system  ${{X}_{1}}f\,=\,{{a}_{1}},\,{{X}_{2}}f=\,{{a}_{2}},\,{{X}_{3}}f\,=\,{{a}_{3}},\,\text{and}\,{{X}_{4}}f\,=\,{{a}_{4}}$. In addition, a formula for the solution function
${{X}_{1}}f\,=\,{{a}_{1}},\,{{X}_{2}}f=\,{{a}_{2}},\,{{X}_{3}}f\,=\,{{a}_{3}},\,\text{and}\,{{X}_{4}}f\,=\,{{a}_{4}}$. In addition, a formula for the solution function 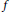 $f$ is deduced, assuming solvability of the system.
$f$ is deduced, assuming solvability of the system.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2018
References
[1]
Calin, O., Chang, D. C., and Eastwood, M., Integrability conditions for Heisenberg and Grushin-type distributions. Anal. Math. Phys. 4(2014), no. 1-2, 99–114. http://dx.doi.Org/10.1007/s13324-014-0073-1Google Scholar
[2]
Calin, O., Chang, D. C., and Hu, J., Poincare's lemma on the Heisenberg group. Adv. in Appl. Math. 60(2014), 90–102. http://dx.doi.org/10.101 6/j.aam.2014.08.003Google Scholar
[3]
Chang, D. C., Markina, I., and Wang, W., On the Cauchy-Szegö kernet for quaternion Siegel upper half-space. Complex Anal. Oper. Theory 7(2013), no. 5, 1623–1654. http://dx.doi.Org/10.1007/s11785-012-0282-2Google Scholar
[4]
Chow, W.-L., Über Systeme von linearen partiellen Differentialgleichungen erster Ordnung. Math. Ann. 117(1939), 98–105. http://dx.doi.Org/10.1007/BF01450011Google Scholar
[5]
Fefferman, C. and Phong, D. H., The uncertainty principle and sharp Garding inequalities. Comm. Pure and Applied Math. 34(1981), 285–331. http://dx.doi.org/10.1002/cpa.3160340302Google Scholar
[6]
Hörmander, L., Hypoelliptic second order differential equations. Acta Math. 119(1967), 147–171. http://dx.doi.org/10.1007/BF02392081Google Scholar
[7]
Wang, W., The tangential Cauchy-Fueter complex on the quaternionic Heisenberg group. J. Geom. Phys. 61(2011), 363–380. http://dx.doi.Org/10.1016/j.geomphys.2010.10.006Google Scholar


