Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-12-01T18:25:15.416Z Has data issue: false hasContentIssue false
  • English
  • Français

Oscillation on Finite or Infinite Intervals of Second Order Linear Differential Equations(1)

Published online by Cambridge University Press:  20 November 2018

D. Willett
D. Willett*
Affiliation:
University of Alberta, Edmonton, Alberta; University of Utah, Salt Lake City
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Recently, Ronveaux [11] has shown how to use a combination of a Riccati transformation and a homographie transformation to estimate both from below and above the distance between a zero and the succeeding or preceding extremum (zero of y' ) of solutions of

1.1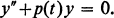

In this paper, we show how such transformations can be used to derive an equation from which the distance between successive zeros of a solution y of (1.1) can be estimated directly.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 14 , Issue 4 , December 1971 , pp. 539 - 550
Copyright
Copyright © Canadian Mathematical Society 1971

Footnotes

(1)

Research supported, in part, by National Research Council of Canada Grant A3053 to the Univ. of Alberta.

References

1. Banks, D., Bounds for eigenvalues and generalized convexity, Pacific J. Math. 13 (1963), 1031-1052.Google Scholar
2. Elbert, A., On the solutions of the differential equation y"+q(x)y = 0, where [q(x)]γ is concave, II, Studia Sci. Math. Hung. 4 (1969), 257-266.Google Scholar
3. Fink, A. M., On the zeros of y"+py = Q with linear, convex, and concave p, J. Math. Pures Appl. 46 (1967), 1-10.Google Scholar
4. Fink, A. M. and Mary, D. F. St., On an inequality of Nehari, Proc. Amer. Math. Soc. 21 (1969), 640-642.Google Scholar
5. Galbraith, A., On the zeros of solutions of ordinary differential equations of the second order, Proc. Amer. Math. Soc. 17 (1966), 333-337.Google Scholar
6. Hartman, P. and Wintner, A., On an oscillation criterion of Lyapunov, Amer. J. Math. 73 (1951), 885–890.Google Scholar
7. Hartman, P. and Wintner, A., On an oscillation criterion of de la Vallée Poussin, Quart. Appl. Math. 13 (1955), 330-332.Google Scholar
8. Lyapunov, A., Sur une série relative à la théorie des équations différentielles linéaires à coefficient périodiques, C.R. Acad. Sci. Paris, 123 (1896), 1248-1252.Google Scholar
9. Opial, Z., Sur les intégrales oscillantes de l'équation différentielle u"+f(t)u = 0, Ann. Polon. Math. 4 (1958), 308-313.Google Scholar
10. Opial, Z., Sur une inégalité de C. de la Vallée Poussin dans la théorie de l'équation différentielle linéaire du second ordre, Ann. Polon. Math. 6 (1959–60), 87-91.Google Scholar
11. Ron veaux, A., Equations différentielles du second ordre: distances entre zéro et extremum des solutions, Ann. Soc. Sci. Bruxelles Sér. I, 84 (1970), 5-20.Google Scholar
12. de la Vallée Poussin, C., Sur l'équation différentielle linéaire du second ordre, J. Math. Pures Appl. 8 (1929), 125-144.Google Scholar
13. Willett, D., Classification of second order linear differential equations with respect to oscillation, Advances in Math. 3 (1969), 594-623.Google Scholar
14. Willett, D., A necessary and sufficient condition for the oscillation of some linear second order differential equations, Rocky Mt. Math. J. 1 (1970), 357-365.Google Scholar