Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T22:06:33.515Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Lattice of Existence Varieties of Locally Inverse Semigroups

Published online by Cambridge University Press:  20 November 2018

Karl Auinger
Karl Auinger*
Affiliation:
Institut für Mathematik Strudlhofgasse 4 A-1090 Wien Austria e-mail:[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

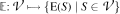The mapping which assigns to each existence variety 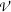of locally inverse semigroups the class of all pseudosemilattices of idempotents of members of 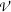is shown to be a complete, surjective homomorphism from the lattice of existence varieties of locally inverse semigroups onto the lattice of varieties of pseudosemilattices.

Keywords

20M07
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 37 , Issue 1 , 01 March 1994 , pp. 13 - 20
Copyright
Copyright © Canadian Mathematical Society 1994

References

1. Auinger, K., The bifree locally inverse semigroup on a set, Algebra, J., to appear.Google Scholar
2. Grätzer, G., Universal Algebra, Van Nostrand, New York, 1968.Google Scholar
3. Hall, T. E., Identities for existence varieties of regular semigroups, Bull. Austral. Math. Soc. 40(1989), 59–77.Google Scholar
4. Hall, T. E., Regular semigroups: amalgamation and the lattice of existence varieties.Algebra Universalis 29(1991), 79–108.Google Scholar
5. Hall, T. E., A concept of variety for regular semigroups, Proceedings 1990 Monash Conference.Google Scholar
6. Kadourek, J. and Szendrei, M. B., A new approach in the theory of orthodox semigroups, Semigroup Forum 40(1990), 257–296.Google Scholar
7. Meakin, J., The free local semilattice on a set, J. Pure Appl. Algebra 27(1983), 263–273.Google Scholar
8. Meakin, J. and Pastijn, F., The structure of pseudosemilattices, Algebra Universalis 13(1981), 355–372.Google Scholar
9. Nambooripad, K. S. S., Structure of regular semigroups, Mem. Amer. Math. Soc. 224(1979).Google Scholar
10. Nambooripad, K. S. S., Pseudosemilattices and biordered sets I, Simon Stevin 55(1981), 103–110.Google Scholar
11. Nambooripad, K. S. S., The natural partial order on a regular semigroup, Proc. Edinburgh Math. Soc. 23(1980), 249–260.Google Scholar
12. Pastijn, F., Rectangular bands of inverse semigroups, Simon Stevin 56(1982), 1–97.Google Scholar
13. Pastijn, F., The structure of pseudo-inverse semigroups, Trans. Amer. Math. Soc. 273(1982), 631–655.Google Scholar
14. Pastijn, F and Petrich, M., Congruences on regular semigroups, Trans. Amer. Math. Soc. 295(1986), 607– 633.Google Scholar
15. Schein, B. M., Pseudosemilattices and pseudolattices, (Russian) Izv. Vyssh. Uchebn. Zaved. Mat. 117 (1972), 81-94; English Trans. Amer. Math. Soc. 119(1983), 1–16.Google Scholar
16. Yeh, Y. T., The existence of e-free objects in e-varieties of regular semigroups, Internat. J. Algebra Comput., 2(1992), 471–484.Google Scholar