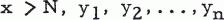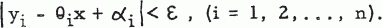Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Widmer, Martin
2018.
WEAK ADMISSIBILITY, PRIMITIVITY, O‐MINIMALITY, AND DIOPHANTINE APPROXIMATION.
Mathematika,
Vol. 64,
Issue. 2,
p.
475.
Jitomirskaya, Svetlana
and
Liu, Wencai
2019.
Inhomogeneous Diophantine approximation in the coprime setting.
Advances in Mathematics,
Vol. 355,
Issue. ,
p.
106773.
Moshchevitin, Nikolay G.
2022.
On the distribution of nonprimitive lattice points in the plane.
Canadian Mathematical Bulletin,
Vol. 65,
Issue. 1,
p.
198.
Moshchevitin, Nikolay
2023.
Einige Bemerkungen über inhomogene diophantische Approximationen.
Archiv der Mathematik,
Vol. 120,
Issue. 2,
p.
159.