Hostname: page-component-f554764f5-8cg97 Total loading time: 0 Render date: 2025-04-12T10:52:56.720Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Convergence Vector Space ℒ,(E, F) and its Dual Space

Published online by Cambridge University Press:  20 November 2018

Ronald Beattie
Ronald Beattie*
Affiliation:
Universitát Mannheim, Lehrstuhl für Mathematik I, 68 Mannheim, Schloss, Germany
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let E be a locally convex tvs, F a normed space and 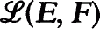the space of continuous linear mappings from E into F In this paper, we investigate the continuous convergence structure (c-structure) on. denotes the resulting convergence vector space (cvs).

The c-structure is by definition the coarsest cvs structure on 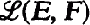making evaluation a continuous mapping.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 21 , Issue 3 , 01 September 1978 , pp. 279 - 284
Copyright
Copyright © Canadian Mathematical Society 1978

References

1. Binz, E., Continuous Convergence on , Lecture Notes in Mathematics, no. 469, Springer-Verlag, Berlin, 1975.Google Scholar
2. Butzmann, H. P., Über die -Reflexivität von , Comment. Math. Helv., 47 (1972), 92-101.Google Scholar
3. Fischer, H. R., Limesräume, Math. Ann., 137 (1959), 269-303.Google Scholar
4. Grothendieck, A., Produits Tensoriels Topologiques et Espaces Nucléaires, Memoirs of the A.M.S. 16, 1966.Google Scholar
5. Schaefer, H. H., Topological Vector Spaces, Springer-Verlag, 1971.Google Scholar