Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-03T19:40:47.067Z Has data issue: false hasContentIssue false
  • English
  • Français

On Singular Normal Linear Integral Equations

Published online by Cambridge University Press:  20 November 2018

Charles G. Costley
Charles G. Costley*
Affiliation:
McGill University, Montreal, Quebec
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this work we consider the equation

1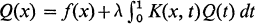

where K(x, y) is singular in the sense that it does not properly belong to L2 and f(x) is an arbitrary L2 function.

A Lebesgue measurable function K(x, y) of two variables, having real values on [0.1] × [0.1] is called a singular normal kernel of

  1. (i)

    There exists approximating kernels Km(x, y) satisfying

  2. (ii)

  3. (iii)

  4. (iv)

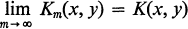
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 13 , Issue 2 , June 1970 , pp. 199 - 203
Copyright
Copyright © Canadian Mathematical Society 1970

References

1. Goldfain, I. A., On a class of linear integral equations, Transi. Amer. Math. Soc. (2) 10 (1958), p. 283.Google Scholar
2. Carleman, T., Sur les équations intégrales singulières à Noyau Riel et symétrique, Uppsala, 1923.Google Scholar
3. Riesz, M. F., Uber Système Integrierbarer Funktionen, Math. Ann., 1910.Google Scholar
4. Stone, M. H., Linear transformation in Hilbert space, Amer. Math. Soc., Coll. XV, New York, 1932.Google Scholar
5. Trjitzinsky, W. J., Problems in the theory of integral equations, Ann. of Math., 1940.Google Scholar