No CrossRef data available.
Article contents
On Locating Isometric ๐(n)1
Published online by Cambridge University Press:ย 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the โSave PDFโ action button.
Motivated by a question of Per Enflo, we develop a hypercube criterion for locating linear isometric copies of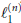 
$\ell _{1}^{\left( n \right)}$ in an arbitrary real normed space 
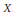$X$.
The said criterion involves finding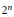 
${{2}^{n}}$ points in 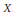$X$ that satisfy one metric equality. This contrasts nicely to the standard classical criterion wherein one seeks 
$n$ points that satisfy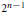 
${{2}^{n-1}}$ metric equalities.
- Type
- Research Article
- Information
- Copyright
- Copyright ยฉ Canadian Mathematical Society 2001
References
[BMW]
Bourgain, J., Milman, V. and Wolfson, H., On type of metric spaces. Trans. Amer.Math. Soc. 294 (1986), 295โ317.Google Scholar
[DL]
Deza, M. M. and Laurent, M., Geometry of Cuts and Metrics. Algorithms and Combinatorics 15(1997), Springer Verlag, 1โ600.Google Scholar
[E]
Enflo, P., On the non-existence of uniform homeomorphisms between Lp-spaces. Ark.Mat. 8 (1969), 103โ105.Google Scholar
[LTW1]
Lennard, C., Tonge, A. and Weston, A., Generalized roundness and negative type. Michigan J. Math. 44 (1997), 37โ45.Google Scholar
[LTW2]
Lennard, C., Tonge, A. and Weston, A., Roundness and metric type. J. Math. Anal. Appl. (to appear in 2000), 1โ10.Google Scholar
[L]
Lรถvblom, G.-M., Uniform homeomorphisms between unit balls in Lp-spaces, Math. Scand. 62 (1988), 294โ302.Google Scholar
[P]
Pisier, G., Probabilistic Methods in the Geometry of Banach Spaces. Springer LectureNotes in Math. 1206 (1985), 1โ75.Google Scholar
You have
Access