Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-26T19:35:52.832Z Has data issue: false hasContentIssue false
  • English
  • Français

On Closed Ideals in a Certain Class of Algebras of Holomorphic Functions

Published online by Cambridge University Press:  20 November 2018

Héctor Merino-Cruz  and
Antoni Wawrzyńczyk
Héctor Merino-Cruz
Affiliation:
Universidad Autónoma de Guerrero, Unidad Académica deMatemáticas, Av. Lázaro Cárdenas S/N, Col. La Haciendita, 39127 Chilpancingo, Gro., México. e-mail: [email protected]
Antoni Wawrzyńczyk
Affiliation:
Departamento de Matemáticas, Universidad Autónoma Metropolitana-Iztapalapa AP 55-534 México D. F., México. e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We recently introduced a weighted Banach algebra 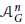$\mathcal{A}_{G}^{n}$ of functions that are holomorphic on the unit disc 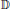$\mathbb{D}$, continuous up to the boundary, and of the class 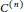${{C}^{\left( n \right)}}$ at all points where the function 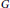$G$ does not vanish. Here, 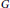$G$ refers to a function of the disc algebra without zeros on 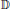$\mathbb{D}$. Then we proved that all closed ideals in 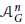$\mathcal{A}_{G}^{n}$ with at most countable hull are standard. In this paper, on the assumption that 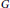$G$ is an outer function in 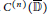${{C}^{\left( n \right)}}\,\left( {\bar{\mathbb{D}}} \right)$ having infinite roots in 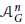$\mathcal{A}_{G}^{n}$ and countable zero set 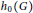${{h}_{o}}\left( G \right)$, we show that all the closed ideals $I$ with hull containing 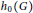${{h}_{o}}\left( G \right)$ are standard.

Keywords

46J1546J2030H50Banach algebradisc algebraholomorphic spacesstandard ideal
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 58 , Issue 2 , 01 June 2015 , pp. 350 - 355
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Gale, J. E., Miana, P. J., and Royo, J. J., Estimates of the Laplace transform on convolution Sobolev algebras. J. Approx. Theory 164 (2012), no. 1,162–178. http://dx.doi.Org/10.1016/j.jat.2O11.09.011 Google Scholar
[2] Gale, J. E. and Wawrzynczyk, A., Standard ideals in weighted algebras of Korenblyum and Wiener types. Math. Scand. 108 (2011), no. 2, 291–319.Google Scholar
[3] Hoffman, K., Banach spaces of analytic functions. Prentice-Hall Series in Modern Analysis, Prentice Hall, Englewood Cliffs, NJ, 1962.Google Scholar
[4] Korenblyum, B. I., Closed ideals in the ring A”. Funkcional. Anal, i Prilozen. 6 (1972), no. 3, 38–52.Google Scholar
[5] Merino-Cruz, H. and Wawrzynczyk, A., Closed ideals in a new class of algebras of holomorphic functions on the disc. Comment. Math. 54 (2014), no. 1, 29–37.Google Scholar
[6] Soltysiak, A. and Wawrzynczyk, A., Ditkin's condition and ideals with at most countable hull in algebras of functions analytic in the unit disc. Comment. Math. 52 (2012), no. 1,101–112.Google Scholar