Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-30T20:45:10.236Z Has data issue: false hasContentIssue false
  • English
  • Français

On a Topology Generated by Measurable Covers

Published online by Cambridge University Press:  20 November 2018

W. Eames
W. Eames*
Affiliation:
Lakehead University, Thunder Bay, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In [2] we showed how, for a certain class of outer measures on a metric space, a measurable cover 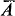could be constructed for each subset A of the space. The function is a closure operator, and in this note some of the properties of the resulting topology are investigated. In particular, we obtain a sufficient condition for the space to be connected.

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 14 , Issue 4 , December 1971 , pp. 499 - 502
Copyright
Copyright © Canadian Mathematical Society 1971

References

1. Cantor, R., Eisenberg, M. and Mandelbaum, E. M., A theorem on Riemann integration, J. London Math. Soc. 37 (1962), 285-286.Google Scholar
2. Eames, W., A Local Property Of Measurable Sets, Canad. J. Math. 12 (1960), 632-640.Google Scholar
3. Eames, W. and May, L. E., Measurable cover functions, Canad. Math. Bull. 10 (1967), 519-523.Google Scholar
4. Goffman, C. and Waterman, D., Approximately continuous transformations, Proc. Amer. Math. Soc. 12 (1961), 116-121.Google Scholar
5. Martin, N. F. G., A topology for certain measure spaces, Trans. Amer. Math. Soc. 112 (1964), 1-18.Google Scholar
6. Troyer, R. S. and Ziemer, W. P., Topologies generated by outer measures, J. Math. Mech. 12 (1963), 485-494.Google Scholar