No CrossRef data available.
Article contents
A Note on Toric Varieties Associated with Moduli Spaces
Published online by Cambridge University Press: 20 November 2018
Abstract
In this note we give a brief review of the construction of a toric variety 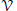 $\mathcal{V}$ coming from a genus
$\mathcal{V}$ coming from a genus 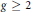 $g\,\ge \,2$ Riemann surface
$g\,\ge \,2$ Riemann surface 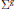 ${{\sum }^{g}}$ equipped with a trinion, or pair of pants, decomposition. This was outlined by J. Hurtubise and L. C. Jeffrey. A. Tyurin used this construction on a certain collection of trinion decomposed surfaces to produce a variety
${{\sum }^{g}}$ equipped with a trinion, or pair of pants, decomposition. This was outlined by J. Hurtubise and L. C. Jeffrey. A. Tyurin used this construction on a certain collection of trinion decomposed surfaces to produce a variety  $D{{M}_{g,}}$ the so-called Delzant model of moduli space, for each genus
$D{{M}_{g,}}$ the so-called Delzant model of moduli space, for each genus  $g$. We conclude this note with some basic facts about the moment polytopes of the varieties
$g$. We conclude this note with some basic facts about the moment polytopes of the varieties 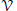 $\mathcal{V}$. In particular, we show that the varieties
$\mathcal{V}$. In particular, we show that the varieties  $D{{M}_{g}}$ constructed by Tyurin, and claimed to be smooth, are in fact singular for
$D{{M}_{g}}$ constructed by Tyurin, and claimed to be smooth, are in fact singular for 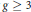 $g\,\ge \,3$.
$g\,\ge \,3$.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2011


