Hostname: page-component-745bb68f8f-g4j75 Total loading time: 0 Render date: 2025-01-12T13:54:38.633Z Has data issue: false hasContentIssue false
  • English
  • Français

A Note on Combinations

Published online by Cambridge University Press:  20 November 2018

M. Abramson  and
W. Moser
M. Abramson
Affiliation:
McGill University
W. Moser
Affiliation:
McGill University
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We call k integers x1 < x2 … < xk chosen from 1, 2, …, n} a k-choice (combination) from n. With 1, 2, …, n arranged in a circle, so that 1 and n are consecutive, we have a circular k-choice from n. A part of a k-choice from n is a sequence of consecutive integers not contained in a longer one. Let 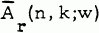denote the number of circular k-choices from n with exactly r parts all ≤ w.

Type
Notes and Problems
Information
Canadian Mathematical Bulletin , Volume 9 , Issue 5 , December 1966 , pp. 675 - 677
Copyright
Copyright © Canadian Mathematical Society 1966

References

1. Abramson, M., Restricted choices. Canad. Math. Bull. 8 (1965) 585-600.Google Scholar
2. Abramson, M. and Moser, W., Combinations, successions and the n-kings problem. Math. Mag.Google Scholar
3. Riordan, J., Permutations without 3-sequences. Bull. Amer. Math. Soc. 51 (1945) 745-48.Google Scholar
4. Riordan, J., An Introduction to Combinatorial Analysis. New York, 1958.Google Scholar